Lorentz transformation

A coordinate transformation that connects two Galilean coordinate systems (cf. Galilean coordinate system) in a pseudo-Euclidean space; in other words, a Lorentz transformation preserves the square of the so-called interval between events. A Lorentz transformation is an analogue of an orthogonal transformation (or a generalization of the concept of a motion) in Euclidean space. The Lorentz transformations form a group, called the Lorentz group (or the general Lorentz group), which is denoted by 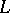. Lorentz transformations find applications in the four-dimensional space-time of the special theory of relativity, for which in Galilean coordinates , , , 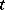the interval has the form

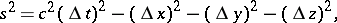where is the velocity of light in vacuum.

One often considers more restricted classes of Lorentz transformations. Thus, the Lorentz transformations that preserve the sign of the coordinate 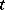form the so-called orthochronous Lorentz group 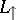. The Lorentz transformations whose matrices have positive determinants are called proper Lorentz transformations and form the proper Lorentz group 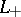. The intersection of 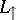and 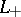is often simply called the Lorentz group.

The general Lorentz group consists of combinations of spatial reflections, reflections in time, spatial rotations, and transformations that from the physical point of view are the transformations of transition from one inertial reference system to another, moving with respect to the first with velocity 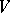, and from the mathematical point of view are hyperbolic rotations through an angle 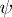in a plane with a pseudo-Euclidean metric. The existence of the latter type of transformations is a specific feature of the group of Lorentz transformations.

For the transition from the Galilean coordinate system 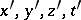to the Galilean coordinate system 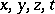, moving with respect to the first with velocity parallel to the -axis, these transformations have the form

If one introduces the angle of hyperbolic rotation 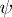by the formulas

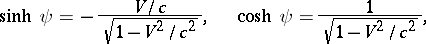then the Lorentz transformations take the form

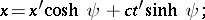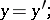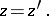These transformations are often simply called Lorentz transformations. They do not form a group: the action of three hyperbolic rotations with non-parallel velocity vectors may give an ordinary spatial rotation, the so-called Thomas precession.

One often supplements the general Lorentz transformations with displacements of the origin, thus obtaining the so-called Poincaré transformations, which form the Poincaré group.

The properties of the group of Lorentz transformations are similar to the properties of the orthogonal groups (cf. Orthogonal group). The differences are connected with the existence of two types of reflections (space and time) and with the fact that the group of Lorentz transformations is not compact (since the unit sphere in a pseudo-Euclidean space, that is, the set of points for which the modulus of the interval up to the origin is equal to one, is not compact).

The physical applications of Lorentz transformations are connected with Einstein's relativity principle, according to which all physical laws, except the law of gravitation, are invariant under Lorentz transformations. In a number of cases, for example in axiomatic quantum field theory, the use of this and other equally general postulates makes it possible to make far-reaching deductions about the forms of functional dependencies between different physical quantities.

In various branches of physics (particularly in the theory of elementary particles) representations of both the homogeneous Lorentz group and the Poincaré group find wide application. In accordance with Einstein's relativity principle physical quantities with different transformation properties — vectors, spinors, tensors — are transformed according to various representations of the Lorentz group. It turns out that relevant unitary representations of the Poincaré group can be characterized by two invariants, to be identified with mass and spin of the particles, the quantum-mechanical states of which transform according to the unitary representation in question.

Infinitesimal Lorentz transformations, that is, rotations through an infinitesimal angle, are often used to obtain various conservation laws.

There are also applications of Lorentz transformations in the tangent space of a pseudo-Riemannian space; these transformations are related to the so-called local space-time symmetries.

Lorentz transformations take their name from the works of H. Lorentz in electron theory, which have played an important role in the formulation of this theory.

References

[1]	E.M. Lifschits, "The classical theory of fields" , Pergamon (1975) (Translated from Russian)
[2]	M.A. Naimark, "Les répresentations linéaires du groupe de Lorentz" , Dunod (1962) (Translated from Russian)
[3]	, [Soviet] Physical Encyclopedic Dictionary , 3 , Moscow (1963) (In Russian)

References

[a1]	E.P. Wigner, "Unitary representations of the inhomogeneous Lorentz group including reflections" , Istambul Summer School of Theoretical Physics, 1962 , Gordon & Breach (1964) pp. 37–80
[a2]	A.S. Wightman, "L'invariance dans la mécanique quantique relativiste" , Ecole d'Eté de Physique Théorique: LesHouches, 1960 , Hermann & Wiley (1960) pp. 159–226
[a3]	W. Ruhl, "The Lorentz group and harmonic analysis" , Benjamin (1970)
[a4]	R.U. Saxl, H.K. Urbantke, "Relativität, Gruppen, Teilchen" , Springer (1976)