Holonomy group

A characteristic of a connection on a fibre bundle. The holonomy group is defined for a principal fibre bundle with a Lie structure group and (second countable) base on which an infinitesimal connection is given. It is also defined for any fibre bundle associated to whose fibres are copies of some representation space of .

The connection on 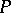(or, respectively, on ) defines, for any piecewise-smooth curve in , an isomorphic mapping between the fibres corresponding to the beginning and the end of . To each piecewise-smooth closed curve in beginning and ending at a point 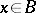corresponds an automorphism of the fibre (or, respectively, ) over the point . These automorphisms form a Lie group , which is called the holonomy group of the connection at .

If the base is (pathwise) connected, then 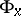and are isomorphic for any and in . One may accordingly speak of the holonomy group of a bundle (or 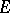) with connection and with (pathwise) connected base.

The holonomy group is a subgroup of the structure group . In the case of a linear connection on 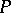this subgroup may be defined directly. Let a point 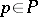in the fibre 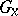over a point be given. The set of elements 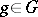such that the points and can be connected by horizontal curves in forms a subgroup of , which is isomorphic to .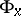

The limited (restricted) holonomy group is the subgroup of the holonomy group generated by the closed curves that are homotopic to zero. It coincides with the pathwise-connected component of the unit element of ; moreover, is at most countable.

The role of holonomy groups in the differential geometry of fibre bundles is explained by the following theorems on connections on .

Reduction theorem. Let be a principal fibre bundle satisfying the second axiom of countability; let 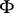be the holonomy group of the connection defined on 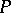. Then the structure group 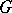is reducible to its subgroup , and the connection is reducible to a connection on the reduced fibre bundle , the holonomy group of which coincides with .

Holonomy theorem. The holonomy algebra (the algebra of the restricted holonomy group) is a subalgebra of the algebra of 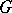generated by all vectors 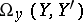, where is the curvature form at the point , where 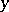runs through the set of points which may be connected with the beginning point by a horizontal path, an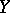d and 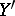are arbitrary horizontal vectors.

References

[1]	D. Gromoll, W. Klingenberg, W. Meyer, "Riemannsche Geometrie im Grossen" , Springer (1968)
[2]	R.L. Bishop, R.J. Crittenden, "Geometry of manifolds" , Acad. Press (1964)
[3]	S. Sternberg, "Lectures on differential geometry" , Prentice-Hall (1964)