Topological structure (topology)

open topology, respectively, closed topology

A collection , respectively , of subsets of a set , satisfying the following properties: 1) The set , as well as the empty set , are elements of the collection , respectively .

, respectively . The intersection, respectively union, of a finite number, and the union, respectively intersection, of any number of elements of , respectively , is an element of the same collection.

Once a topology, or topological structure, has been introduced or defined on a set , the set is called a topological space, its elements are called points and the elements of the collection 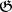, respectively , are called the open, respectively closed, sets of this topological space.

If one of the collections or of subsets of is defined, satisfying property 1 and or , respectively, then the other collection may be defined by duality as consisting of the complements of the elements of the first collection.

Comments

See also Topology, general; Topological space; General topology.

References

[a1]	R. Engelking, "General topology" , Heldermann (1989)