Maximal invariant

An invariant statistic that takes different values on the different orbits generated by a group of one-to-one measurable transformations of the sampling space. Thus, if 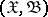is a sampling space and 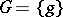is a group of one-to-one 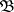-measurable transformations of 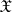onto itself, then an invariant statistic 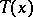is a maximal invariant if 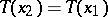implies that 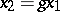for some 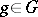. For example, if 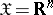, , 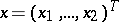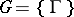is the group of orthogonal transformations 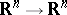, and 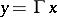, then the statistic 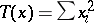is a maximal invariant. Any invariant statistic is a function of the maximal invariant.

Maximal invariants are used for the construction of invariant tests (cf. Invariant test).

References

[1]	E.L. Lehmann, "Testing statistical hypotheses" , Wiley (1959)
[2]	S. Zacks, "The theory of statistical inference" , Wiley (1975)
[3]	G.P. Klimov, "Invariant inferences in statistics" , Moscow (1973) (In Russian)