Betti number
From Encyclopedia of Mathematics
 -dimensional Betti number
-dimensional Betti number  of a complex
of a complex 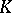
The rank of the  -dimensional Betti group with integral coefficients. For each
-dimensional Betti group with integral coefficients. For each  the Betti number
the Betti number 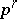 is a topological invariant of the polyhedron which realizes the complex
is a topological invariant of the polyhedron which realizes the complex  , and it indicates the number of pairwise non-homological (over the rational numbers) cycles in it. For instance, for the sphere
, and it indicates the number of pairwise non-homological (over the rational numbers) cycles in it. For instance, for the sphere 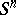 :
:
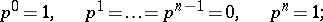 |
for the projective plane 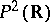 :
:
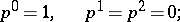 |
for the torus  :
:
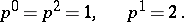 |
For an  -dimensional complex
-dimensional complex 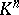 the sum
the sum
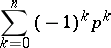 |
is equal to its Euler characteristic. Betti numbers were introduced by E. Betti [1].
References
| [1] | E. Betti, Ann. Mat. Pura Appl. , 4 (1871) pp. 140–158 |
Comments
References
| [a1] | E.H. Spanier, "Algebraic topology" , McGraw-Hill (1966) |
How to Cite This Entry:
Betti number. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Betti_number&oldid=32659
Betti number. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Betti_number&oldid=32659
This article was adapted from an original article by M.I. Voitsekhovskii (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article