Factor algebra

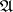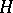An involutive subalgebra of the algebra of linear operators on a Hilbert space that is closed relative to so-called weak convergence of operators and has the property that its centre (that is, the collection of all operators in 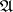that commute with every operator in 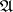) consists of scalar multiples of the unit operator.

If 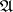is a factor, then for a large supply of subspaces of one can define the concept of the dimension 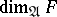relative to 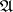as an invariant that is preserved, not under arbitrary isometries 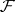, but only under those in the given factor with additional natural properties (for example, 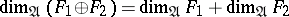). All factors can be divided into five classes corresponding to the values that 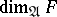can take, where, for example, for a factor of class 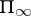it can take any value in .

Comments

An involutive algebra is an algebra over 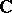endowed with an involution. For information concerning various types of factors cf. von Neumann algebra.

References

[a1]	G.K. Pedersen, "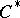-algebras and their automorphism groups" , Acad. Press (1979)
[a2]	J. Dixmier, "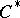 algebras" , North-Holland (1977) (Translated from French)