Arithmetic series
of order 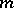
The sequence of values of a polynomial of degree  :
:
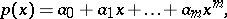 |
assumed by the polynomial when the variable  takes successive integral non-negative values
takes successive integral non-negative values 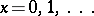 . If
. If  , i.e.
, i.e. 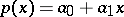 , one obtains an arithmetic progression with initial term
, one obtains an arithmetic progression with initial term 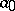 and difference
and difference  . If
. If 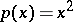 or
or 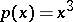 , one obtains sequences of squares or cubes of integers, i.e. special cases of arithmetic series of the second and third orders. If a first difference series is created, constituted by the differences between successive terms of an arithmetic series, then a series of differences of the first difference series (second differences) is written, and then the second differences are used to form third differences, etc., one finds, at the
, one obtains sequences of squares or cubes of integers, i.e. special cases of arithmetic series of the second and third orders. If a first difference series is created, constituted by the differences between successive terms of an arithmetic series, then a series of differences of the first difference series (second differences) is written, and then the second differences are used to form third differences, etc., one finds, at the 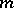 -th stage, that all
-th stage, that all 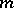 -th differences are equal. Conversely, if the
-th differences are equal. Conversely, if the 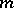 -th differences of a numerical sequence are all equal, the sequence is an arithmetic series of order
-th differences of a numerical sequence are all equal, the sequence is an arithmetic series of order 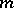 . Using this property, it is possible to construct arithmetic series of different orders from their differences. For example, the sequence
. Using this property, it is possible to construct arithmetic series of different orders from their differences. For example, the sequence 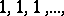 may be regarded as the first differences of the series of natural numbers
may be regarded as the first differences of the series of natural numbers 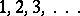 ; as the second differences of the series of triangular numbers
; as the second differences of the series of triangular numbers 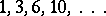 ; as the third differences of the sequence of tetrahedral numbers
; as the third differences of the sequence of tetrahedral numbers 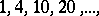 etc. These numbers are so called because triangular numbers represent numbers arranged in the form of a triangle (Fig. a), while tetrahedral numbers represent numbers arranged in the form of tetrahedra (pyramids) (Fig. b).
etc. These numbers are so called because triangular numbers represent numbers arranged in the form of a triangle (Fig. a), while tetrahedral numbers represent numbers arranged in the form of tetrahedra (pyramids) (Fig. b).
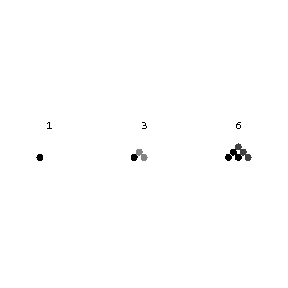
Figure: a013370a
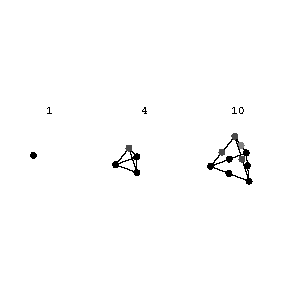
Figure: a013370b
Triangular numbers are expressed by the formula 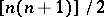 , while tetrahedral numbers are given by the formula
, while tetrahedral numbers are given by the formula
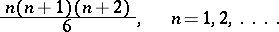 |
A generalization of triangular numbers is constituted by 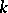 -gonal or figurate numbers, which played an important role in the development of arithmetic in its various stages.
-gonal or figurate numbers, which played an important role in the development of arithmetic in its various stages.

Figure: a013370c

Figure: a013370d
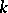 -gonal numbers are given by the formula:
-gonal numbers are given by the formula:
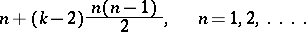 |
They form an arithmetic series of the second order, with one as their first term, 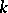 as their second term and
as their second term and 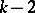 as their second differences. If
as their second differences. If 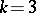 , triangular numbers are obtained; if
, triangular numbers are obtained; if 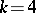 , one obtains squares (
, one obtains squares (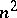 ); if
); if 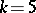 , one obtains pentagonal numbers
, one obtains pentagonal numbers  , etc. These appellations will become clear from Fig. cand Fig. d, in which the number of beads arranged in the form of a square or a pentagon is expressed by the respective square or pentagonal number. Figurate numbers satisfy the following theorem, proposed by P. Fermat and first proved by A.L. Cauchy: Any natural number can be represented as a sum of not more than
, etc. These appellations will become clear from Fig. cand Fig. d, in which the number of beads arranged in the form of a square or a pentagon is expressed by the respective square or pentagonal number. Figurate numbers satisfy the following theorem, proposed by P. Fermat and first proved by A.L. Cauchy: Any natural number can be represented as a sum of not more than 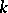
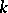 -gonal numbers.
-gonal numbers.
References
| [1] | B.L. van der Waerden, "Algebra" , 1–2 , Springer (1967–1971) (Translated from German) |
| [2] | V.I. Arnol'd, "Theoretical arithmetics" , Moscow (1939) (In Russian) |
Arithmetic series. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Arithmetic_series&oldid=32647