Denjoy integral

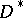The narrow (special) Denjoy integral is a generalization of the Lebesgue integral. A function is said to be integrable in the sense of the narrow (special, ) Denjoy integral on 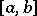if there exists a continuous function on 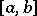such that 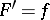almost everywhere, and if for any perfect set 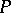there exists a portion of 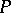on which 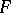is absolutely continuous and where

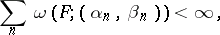where 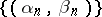is the totality of intervals contiguous to that portion of 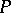and 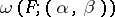is the oscillation of 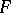on ;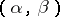

This generalization of the Lebesgue integral was introduced by A. Denjoy

who showed that his integral reproduces the function with respect to its pointwise finite derivative. The 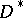integral is equivalent to the Perron integral.

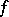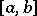The wide (general) Denjoy integral is a generalization of the narrow Denjoy integral. A function is said to be integrable in the sense of the wide (general, ) Denjoy integral on if there exists a continuous functi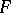on on 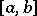such that its approximate derivative is almost everywhere equal to 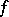and if, for any perfect set 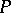, there exists a portion of 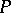on which 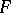is absolutely continuous; here

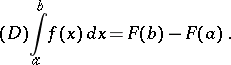Introduced independently, and almost at the same time, by Denjoy

and A.Ya. Khinchin , . The 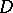integral reproduces a continuous function with respect to its pointwise finite approximate derivative.

A totalization 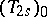is a constructively defined integral for solving the problem of constructing a generalized Lebesgue integral which would permit one to treat any convergent trigonometric series as a Fourier series (with respect to this integral). Introduced by Denjoy .

A totalization 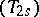differs from a totalization 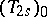by the fact that the definition of the latter totalization involves an approximate rather than an ordinary limit. Denjoy [5] also gave a descriptive definition of a totalization 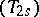. For relations between 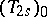and 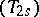and other integrals, see [6].

References

[1a]	A. Denjoy, "Une extension de l'integrale de M. Lebesgue" C.R. Acad. Sci. , 154 (1912) pp. 859–862
[1b]	A. Denjoy, "Calcul de la primitive de la fonction dérivée la plus générale" C.R. Acad. Sci. , 154 (1912) pp. 1075–1078
[2]	A. Denjoy, "Sur la dérivation et son calcul inverse" C.R. Acad. Sci. , 162 (1916) pp. 377–380
[3]	A.Ya. [A.Ya. Khinchin] Khintchine, "Sur une extension de l'integrale de M. Denjoy" C.R. Acad. Sci. , 162 (1916) pp. 287–291
[4]	A.Ya. Khinchin, "On the process of Denjoy integration" Mat. Sb. , 30 (1918) pp. 543–557 (In Russian)
[5]	A. Denjoy, "Leçons sur le calcul des coefficients d'une série trigonométrique" , 1–4 , Gauthier-Villars (1941–1949)
[6]	I.A. Vinogradova, V.A. Skvortsov, "Generalized Fourier series and integrals" J. Soviet Math. , 1 (1973) pp. 677–703 Itogi Nauk. Mat. Anal. 1970 (1971) pp. 65–107
[7]	S. Saks, "Theory of the integral" , Hafner (1952) (Translated from French)

Comments

Just as the Lebesgue integral allows one to compute the mass corresponding to some density function, the Denjoy integral (called totalization by Denjoy also in the case 1) or 2)) allows one to compute the primitive (defined up to a constant) of some function. And, whereas for smooth functions calculating primitives is the usual way of calculating masses, in the general case the calculus of primitives (in the sense of 1) or 2)) depends on and is more involved than the calculus of masses. Denjoy gave a constructive scheme (one for 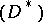and a similar one for ) to calculate when possible the totalization 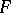of a function 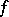by induction over the countable ordinal numbers, something which does not exist for similar integrals like Perron's integral: If has a totalization (for example, if is the derivative in case 1), or the approximate derivative in case 2), of some function) the construction stops at some countable ordinal number and gives 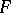; if 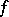does not have a totalization, the construction never stops before 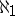. This constructive scheme uses the Lebesgue integral, and two ways of defining "improper" integrals coming from the theory of the Riemann integral for unbounded functions and due, respectively, to A.L. Cauchy and A. Harnack. For details see [7] or [a1].

References

[a1]	G. Choquet, "Outils topologiques et métriques de l'analyse mathématique" , Centre Docum. Univ. Paris (1969) (Rédigé par C. Mayer)