Wedge (in a vector space)

convex cone

A convex set in a vector space over an ordered field that is invariant with respect to the transformation 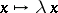, , 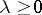that is, a set 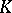such that if 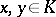and , then 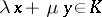. A convex cone 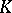satisfying the condition: If 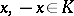then 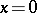, is called a proper (convex) cone. Each proper cone induces in the vector space a quasi-order: 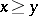when 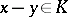. A proper cone 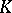in a space 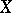is called reproducing (or a domain of positivity) if .

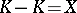Comments

Open sets 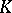defined, as above, by the requirements that 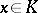implies 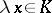, for all 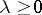, and implies 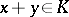, are usually called (open) cones.

Let 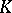be an open cone in a real topological vector space 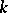, let 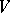be the intersection of 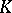with a bounded open ball with centre at 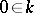, and let 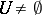be an open set in 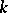. Let

be sets in the complexification of (). Then and 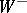are called wedges (and 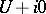is their "edge" ). For a use of wedges in the theory of analytic continuation see Bogolyubov theorem.