Meusnier theorem
From Encyclopedia of Mathematics
If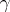 
is a curve lying on a surface and 
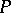is a point on 
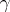, then the curvature 
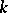of 
at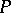 
, the curvature 
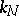of the normal section of the surface by the plane 
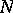passing through the tangent to 
at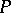 
, and the angle 
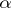between the osculating plane of 
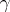at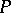 
and the normal plane 
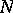, satisfy the relation
 |
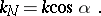In particular, the curvature of every inclined section of the surface can be expressed in terms of the curvature of the normal section with the same tangent.
This theorem was proved by J. Meusnier in 1779 (and was published in [1]).
References
| [1] | J. Meusnier, Mém. prés. par div. Etrangers. Acad. Sci. Paris , 10 (1785) pp. 477–510 |
Comments
References
| [a1] | M.P. Do Carmo, "Differential geometry of curves and surfaces" , Prentice-Hall (1976) pp. 142 |
| [a2] | W. Blaschke, K. Leichtweiss, "Elementare Differentialgeometrie" , Springer (1973) |
How to Cite This Entry:
Meusnier theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Meusnier_theorem&oldid=32553
Meusnier theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Meusnier_theorem&oldid=32553
This article was adapted from an original article by D.D. Sokolov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article