Maximal subgroup
From Encyclopedia of Mathematics
A proper subgroup of a group 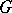 which is not contained in any other proper subgroup of
which is not contained in any other proper subgroup of 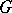 , that is, a maximal element in the set of proper subgroups of
, that is, a maximal element in the set of proper subgroups of 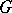 ordered by inclusion. There exist groups without maximal subgroups, for example, a group of type
ordered by inclusion. There exist groups without maximal subgroups, for example, a group of type 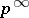 .
.
A generalization of the concept of a maximal subgroup is that of a subgroup maximal with respect to some property 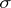 , i.e. a subgroup
, i.e. a subgroup 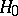 of
of 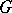 with the property
with the property  and such that no other proper subgroup
and such that no other proper subgroup 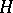 of
of 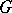 has
has 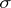 and contains
and contains 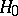 .
.
References
| [1] | M.I. Kargapolov, J.I. [Yu.I. Merzlyakov] Merzljakov, "Fundamentals of the theory of groups" , Springer (1979) (Translated from Russian) |
Comments
A proper subgroup of a group 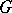 is a subgroup
is a subgroup 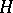 of
of  satisfying
satisfying 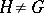 .
.
References
| [a1] | M. Hall jr., "The theory of groups" , Macmillan (1959) |
How to Cite This Entry:
Maximal subgroup. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Maximal_subgroup&oldid=32492
Maximal subgroup. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Maximal_subgroup&oldid=32492
This article was adapted from an original article by N.N. Vil'yams (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article