Functional determinant

A determinant whose elements are functions. Functional determinants of specific types play an important role in mathematical analysis. In the first place this refers to the Jacobian and the Wronskian. The concept of a Jacobian is used in an essential way when studying differentiable mappings between domains of Euclidean spaces , 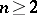; when changing the variable in multiple integrals; when clarifying conditions for determining an implicit function by a system of equations or when a system of given functions is dependent; etc. The concept of the Wronskian is extensively applied in the theory of linear ordinary differential equations.