Montel space
A barrelled space (in particular, a Fréchet space) in which each closed bounded set is compact. The space  of all holomorphic functions in a domain
of all holomorphic functions in a domain 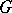 , with the topology of uniform convergence on compact sets, is a Fréchet space and, in view of a theorem of Montel (cf. Montel theorem, 2), every bounded sequence of holomorphic functions is relatively compact in
, with the topology of uniform convergence on compact sets, is a Fréchet space and, in view of a theorem of Montel (cf. Montel theorem, 2), every bounded sequence of holomorphic functions is relatively compact in 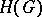 , so
, so 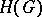 is a Montel space. The space
is a Montel space. The space 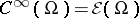 of all infinitely-differentiable functions in a domain
of all infinitely-differentiable functions in a domain 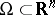 , the space
, the space 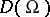 of all functions of compact support and the space
of all functions of compact support and the space 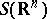 of differentiable functions that are rapidly decreasing at infinity, are also Montel spaces in their natural topologies.
of differentiable functions that are rapidly decreasing at infinity, are also Montel spaces in their natural topologies.
A Montel space is reflexive (cf. Reflexive space). The strong dual of a Montel space is a Montel space; in particular, the spaces of generalized functions  ,
,  and
and 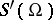 are Montel spaces. A normed space is a Montel space if and only if it is finite-dimensional.
are Montel spaces. A normed space is a Montel space if and only if it is finite-dimensional.
References
| [1] | N. Bourbaki, "Elements of mathematics. Topological vector spaces" , Addison-Wesley (1977) (Translated from French) |
| [2] | A.P. Robertson, W.S. Robertson, "Topological vector spaces" , Cambridge Univ. Press (1964) |
| [3] | R.E. Edwards, "Functional analysis: theory and applications" , Holt, Rinehart & Winston (1965) |
Comments
References
| [a1] | H. Jarchow, "Locally convex spaces" , Teubner (1981) (Translated from German) |
| [a2] | G. Köthe, "Topological vector spaces" , 1 , Springer (1969) |
| [a3] | H.H. Schaefer, "Topological vector spaces" , Springer (1971) |
Montel space. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Montel_space&oldid=32479