Spectral estimator, parametric

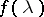An estimator for the spectral density of a stationary stochastic process corresponding to some fixed parametric model of (that is, under the hypothesis that the function 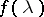belongs to a specific family of spectral densities described by a finite number of parameters). In the determination of parametric spectral estimators, observational data are used only for evaluating the unknown parameters of the model. Consequently, the problem of estimating the spectral density reduces to the statistical problem of estimating these parameters. The most widely used parametric spectral estimator in practice is the maximum-entropy spectral estimator, corresponding to the assumption that the function 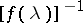is the square of a trigonometric polynomial of fixed order. A more general class of parametric spectral estimators often used in applied problems relies on the use of the mixed autoregressive moving-average process model, that is, on the assumption that is the quotient of the squares of the moduli of two trigonometric polynomials of fixed orders (see [1]–[3]).

References

[1]	S.S. Haykin (ed.) , Nonlinear methods of spectral analysis , Springer (1983)
[2]	S.M. Kay, S.L. Marpl, Trudy Inst. Inzh. Elektrotekhn. Radioelektr. , 69 (1981) pp. 5–51
[3]	"Methods of spectral estimation. Thematic volume" Trudy Inst. Inzh. Elektrotekhn. Radioelektr. , 70 : 9 (1982) (In Russian)

References

[a1]	G.M. Jenkins, D.G. Watts, "Spectral analysis and its applications" , 1–2 , Holden-Day (1968)
[a2]	E.J. Hannan, "Multiple time series" , Wiley (1970)