Normal equation
normalized equation
The equation of a line in a plane of the form
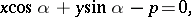 |
where  and
and 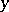 are rectangular Cartesian coordinates in the plane,
are rectangular Cartesian coordinates in the plane, 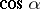 and
and 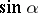 are the coordinates of the unit vector
are the coordinates of the unit vector 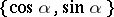 perpendicular to the line, and
perpendicular to the line, and 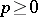 is the distance of the coordinate origin from the line. An equation of a line of the form
is the distance of the coordinate origin from the line. An equation of a line of the form
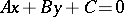 |
reduces to normal form after multiplication by the normalizing factor  of absolute value
of absolute value 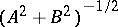 and of sign opposite to that of
and of sign opposite to that of  (if
(if 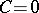 , the sign of
, the sign of 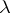 is arbitrary).
is arbitrary).
Similarly, the equation of a plane
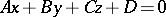 |
reduces to the normal form
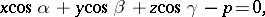 |
where 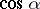 ,
, 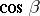 and
and  are the direction cosines of a vector perpendicular to the plane, after multiplication by the normalizing factor
are the direction cosines of a vector perpendicular to the plane, after multiplication by the normalizing factor 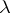 of absolute value
of absolute value 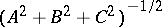 and of sign opposite to that of
and of sign opposite to that of 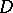 .
.
Normal equation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Normal_equation&oldid=32443