Curve of pursuit
From Encyclopedia of Mathematics
A curve representing a solution of the "pursuit" problem, which is posed as follows: Let a point 
be moved uniformly along a given curve. The trajectory has to be found of the uniform movement of a point 
, such that the tangent drawn towards the trajectory at any moment of the movement would pass through the position of 
corresponding to that moment in time.
Figure: c027400a
In a plane, the system of equations which the curve of pursuit must satisfy takes the form
 |
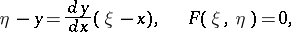where 
is the slope of the curve of pursuit, and 
is the equation of the given curve.
The "pursuit" problem was posed by Leonardo da Vinci and solved by P. Bouguer (1732). For a generalization of it see the last Chapter of [2].
References
| [1] | A.A. Savelov, "Planar curves" , Moscow (1960) (In Russian) |
| [2] | J.E. Littlewood, "A mathematician's miscellany" , Methuen (1953) |
How to Cite This Entry:
Curve of pursuit. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Curve_of_pursuit&oldid=32278
Curve of pursuit. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Curve_of_pursuit&oldid=32278
This article was adapted from an original article by D.D. Sokolov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article