Bianchi surface

A surface of negative Gaussian curvature which can be expressed in asymptotic parameters as

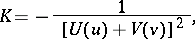where and are arbitrary functions; thus, Bianchi surfaces can be characterized by the fact that the function is diagonal with respect to its asymptotic net, i.e.

For instance, a ruled Bianchi surface is a conoid — a surface attached to the Peterson surface. If a Bianchi surface is given, it is possible to determine the classes of surfaces which can be obtained by a deformation over a principal base and to classify them. Thus, if the principal base contains two families of geodesic lines, the functions and are constant and the bent surface is a Voss surface (the class ).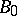

The class 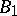is characterized by the fact that only one family of lines of the principal base are geodesics (one of the functions 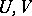is constant); conoids may serve as an example. The class corresponds to functions which both depend non-trivially on their arguments. See also Bianchi congruence.

Comments

The notion of a "deformation over a principal base" is not very common in Western literature, and this type of deformation has no standard name even. It can best be characterized as a deformation preserving a conjugate net.

References

[a1]	L. Bianchi, "Lezioni di geometria differenziale" , 2 , Zanichelli , Bologna (1927) pp. Chapt. 1
[a2]	S.P. Finikov, "Theorie der Kongruenzen" , Akademie Verlag (1959) (Translated from Russian)