Central series of a group
From Encyclopedia of Mathematics
A normal series all factors of which are central, that is, a series of subgroups
 |
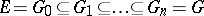for which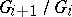 
lies in the centre of 
for all 
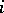(see also Subgroup series). If for all 
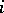the subgroup 
is the complete centre of 
, then the series is called the upper central series of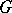 
and if the commutator subgroup of 
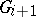and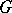 
coincides with 
, then the series is called the lower central series of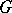 
.
A group having a central series is called a nilpotent group. In a nilpotent group the lower and the upper central series have the same length, which is the minimal length of a central series of the group. This length is called the nilpotency class (or the degree of nilpotency) of the group.
Comments
References
| [a1] | P. Hall, "The theory of groups" , Macmillan (1959) pp. Chapt. 10 |
How to Cite This Entry:
Central series of a group. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Central_series_of_a_group&oldid=31982
Central series of a group. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Central_series_of_a_group&oldid=31982
This article was adapted from an original article by O.A. Ivanova (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article