Osculating paraboloid

of a surface at a point

The paraboloid that reproduces the shape of the surface near this point up to variables of the second order of smallness with respect to the distance from . Let be a paraboloid (see Fig.) with vertex and tangent to the surface at this point, and let and be the distances of an arbitrary point on the paraboloid to the surface and to , respectively.

Figure: o070550a

Then is said to osculate if as . This does not exclude the degeneration of the paraboloid into a parabolic cylinder or plane. At every point of a regular surface there is a unique osculating paraboloid. Osculating paraboloids can be used to classify the points on a surface (see Elliptic point; Hyperbolic point; Parabolic point; Flat point).

Comments

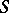The osculating paraboloid at to the surface has contact of order three with at 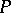, i.e. the derivatives up to and including order 2 of the difference 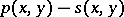of the functions and describing the paraboloid and the surface are all zero at 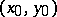, where .

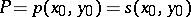References

[a1]	R.S. Millman, G.D. Parker, "Elements of differential geometry" , Prentice-Hall (1977) pp. 138
[a2]	M. Berger, B. Gostiaux, "Differential geometry: manifolds, curves, and surfaces" , Springer (1988) (Translated from French)