Darboux quadric
A second-order surface with second-order contact with a surface 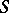 in three-dimensional projective space
in three-dimensional projective space 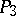 at a point
at a point  , in which the line of intersection with the surface
, in which the line of intersection with the surface 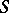 at the point
at the point  has a special type of singularity. Out of the set of quadrics with second-order contact with
has a special type of singularity. Out of the set of quadrics with second-order contact with 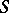 at
at  one can select the quadrics in which the line of intersection with
one can select the quadrics in which the line of intersection with 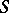 has a singular point
has a singular point  with three coincident tangents. On the surface
with three coincident tangents. On the surface 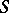 there are three directions (Darboux directions) for these three coincident tangents. At
there are three directions (Darboux directions) for these three coincident tangents. At 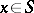 there exists a one-parameter family of Darboux quadrics — the Darboux pencil. A pencil of hyper-quadrics, which are in contact at a point
there exists a one-parameter family of Darboux quadrics — the Darboux pencil. A pencil of hyper-quadrics, which are in contact at a point  with a hypersurface
with a hypersurface 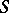 in projective space
in projective space 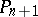 , is an extension of the Darboux pencil. A (non-developable) hypersurface
, is an extension of the Darboux pencil. A (non-developable) hypersurface 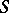 degenerates into a hyper-quadric if and only if its generalized Darboux tensor vanishes [2].
degenerates into a hyper-quadric if and only if its generalized Darboux tensor vanishes [2].
References
| [1] | S.P. Finikov, "Projective-differential geometry" , Moscow-Leningrad (1937) (In Russian) |
| [2] | G.F. Laptev, "Differential geometry of imbedded manifolds. Group theoretical methods of differential geometric investigation" Trudy Moskov. Mat. Obshch. , 2 (1953) pp. 275–382 (In Russian) |
Comments
References
| [a1] | E. Cartan, "Leçons sur la théorie des espaces à connexion projective" , Gauthier-Villars (1937) pp. Part II, Chapt. VI §II |
Darboux quadric. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Darboux_quadric&oldid=31968