Centre of a group

The set of all central elements (sometimes also called invariant elements) of the group, that is, the elements that commute with all elements of the group. The centre of a group is a normal and even a characteristic subgroup in 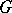. Moreover, every subgroup of the centre is normal in 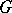. Abelian groups and only these coincide with their centres. Groups whose centres consist only of the unit element are said to be groups without centre or groups with trivial centre. The quotient group 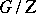of a group by its centre is not necessarily a group without centre.

References

[1]	A.G. Kurosh, "The theory of groups" , 1–2 , Chelsea (1955–1956) (Translated from Russian)