Hausdorff axiom
From Encyclopedia of Mathematics
One of the separation axioms (cf. Separation axiom). It was introduced by F. Hausdorff in 1914 (see [1]) in his definition of the concept of a topological space. The Hausdorff axiom holds in a topological space if any two (distinct) points of it have disjoint neighbourhoods. A space satisfying the Hausdorff axiom is called a Hausdorff space or a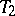 
-space.
References
| [1] | F. Hausdorff, "Set theory" , Chelsea, reprint (1978) (Translated from German) |
Comments
References
| [a1] | A.V. Arkhangel'skii, V.I. Ponomarev, "Fundamentals of general topology: problems and exercises" , Reidel (1984) (Translated from Russian) |
How to Cite This Entry:
Hausdorff axiom. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Hausdorff_axiom&oldid=31890
Hausdorff axiom. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Hausdorff_axiom&oldid=31890
This article was adapted from an original article by I.G. Koshevnikova (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article