Integral domain

integral ring

A commutative ring with identity and without divisors of zero (cf. Divisor). Any field, and also any ring with identity contained in a field, is an integral domain. Conversely, an integral domain can be imbedded in a field. Such an imbedding is given by the construction of the field of fractions (see Fractions, ring of).

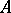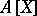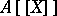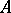If is an integral domain, then the ring of polynomials and the ring of formal power series over are also integral domains. If 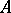is a commutative ring with identity and 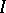is any ideal in 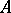, then the ring 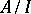is an integral domain if and only if 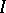is a prime ideal. A ring 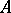without nilpotents is an integral domain if and only if the spectrum of 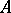is an irreducible topological space (cf. Spectrum of a ring).

Sometimes commutativity of 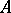is not required in the definition of an integral domain. Skew-fields and subrings of a skew-field containing the identity are examples of non-commutative integral domains. However, it is not true, in general, that an arbitrary non-commutative integral domain can be imbedded in a skew-field (see [2], and Imbedding of rings).

References

[1]	S. Lang, "Algebra" , Addison-Wesley (1965)
[2]	P.M. Cohn, "Free rings and their relations" , Acad. Press (1985)
[3]	C. Faith, "Algebra: rings, modules, and categories" , 1 , Springer (1973)