Vector field

A term which is usually understood to mean a function of points in some space whose values are vectors (cf. Vector), defined for this space in some way.

In the classical vector calculus it is a subset of a Euclidean space that plays the part of , while the vector field represents directed segments applied at the points of this subset. For instance, the collection of unit-length vectors tangent or normal to a smooth curve (surface) is a vector field on it.

If is an abstractly specified differentiable manifold, a vector field is understood to mean a tangent vector field, i.e. a function that associates to each point of an (invariantly constructed) vector tangent to . If is finite-dimensional, the vector field is equivalently defined as a collection of univalent, contravariant tensors, which are depending on the points.

In the general case a vector field is interpreted as a function defined on with values in a vector space associated with in some way; it differs from an arbitrary vector function in that 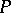is defined with respect to "internally" rather than as a "superstructure" over 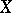. A section of a vector bundle with base is also considered to be a vector field.

Comments

Cf. also Vector field on a manifold.