Leech lattice

A particular lattice (see also Lattice of points; Geometry of numbers) in defined by J. Leech in 1967 [a1] using the close relations between packing of balls and error-correcting binary codes (cf. Error-correcting code), and in particular a code defined by M.J.E. Golay. At present there are many alternative descriptions known. The automorphism group of the Leech lattice (i.e. the group of orthogonal transformations mapping it onto itself) turned out to be of great importance in the search of the sporadic simple groups, see [a2] and Sporadic simple group. 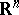The Newton or kissing number of a ball in is the maximum number of non-overlapping balls of the same radius as the given ball that can touch the given ball. Its values are known only for 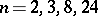, and are 6, 12, 240, and 196.560, respectively. For 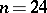this was established by A.M. Odlyzko and N.J.A. Sloane and V.I. Levenshtein independently. The (unique) arrangement for which the Newton number in 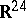is attained is given by a ball packing where the centres belong to the Leech lattice. It has been conjectured that the Leech lattice provides the densest lattice packing of balls in . As a first step towards a verification of this it has been shown that among all lattice packings of balls the Leech lattice ball packing locally has maximum density, cf. [a5]. For a comprehensive survey of the Leech lattice see [a3].

References

[a1]	J. Leech, "Note on sphere packings" Canad. J. Math. , 19 (1967) pp. 251–267
[a2]	R.L. Griess, "The friendly giant" Invent. Math. , 69 (1982) pp. 1–102
[a3]	J.H. Conway, N.J.A. Sloane, "Sphere packing, lattices and groups" , Springer (1988)
[a4]	P.M. Gruber, C.G. Lekkerkerker, "Geometry of numbers" , North-Holland (1987) pp. Sect. (iv) (Updated reprint)
[a5]	P. Erdös, P.M. Gruber, J. Hammer, "Lattice points" , Longman (1989)
[a6]	T.M. Thompson, "From error-correcting codes through sphere packings to simple groups" , Math. Assoc. Amer. (1983)