Condensation point of a set
From Encyclopedia of Mathematics
in a Euclidean space 
A point of 
such that every neighbourhood of it contains uncountable many points of the set. The set of condensation points of a set is always closed; if it is non-empty, it is perfect and has the cardinality of the continuum. The concept of a condensation point can be generalized to arbitrary topological spaces.
Comments
The generalization to arbitrary spaces is direct: A point 
a condensation point (of a set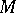 
) in a topological space if (the intersection of 
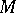with) every neighbourhood of 
is an uncountable set. (See also [a1].)
References
| [a1] | A.V. Arkhangel'skii, V.I. Ponomarev, "Fundamentals of general topology: problems and exercises" , Reidel (1984) (Translated from Russian) |
How to Cite This Entry:
Condensation point of a set. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Condensation_point_of_a_set&oldid=31620
Condensation point of a set. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Condensation_point_of_a_set&oldid=31620
This article was adapted from an original article by B.A. Efimov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article