Irreducible polynomial
A polynomial 
in 
variables over a field 
that is a prime element of the ring 
, that is, it cannot be represented in the form 
where 
and 
are non-constant polynomials with coefficients in 
(irreducibility over 
). A polynomial is called absolutely irreducible if it is irreducible over the algebraic closure of its field of coefficients. The absolutely irreducible polynomials of a single variable are the polynomials of degree 1. In the case of several variables there are absolutely irreducible polynomials of arbitrarily high degree, for example, any polynomial of the form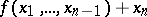 
is absolutely irreducible.
The polynomial ring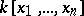 
is factorial (cf. Factorial ring): Any polynomial splits into a product of irreducibles and this factorization is unique up to constant factors. Over the field of real numbers any irreducible polynomial in a single variable is of degree 1 or 2 and a polynomial of degree 2 is irreducible if and only if its discriminant is negative. Over an arbitrary algebraic number field there are irreducible polynomials of arbitrarily high degree; for example, 
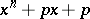, where 
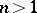and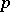 
is a prime number, is irreducible in 
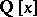by Eisenstein's criterion (see Algebraic equation).
Let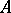 
be an integrally closed ring with field of fractions 
and let 
be a polynomial in a single variable with leading coefficient 1. If 
in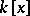 
and both 
and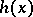 
have leading coefficient 1, then 
(Gauss' lemma).
Reduction criterion for irreducibility. Let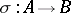 
be a homomorphism of integral domains. If 
and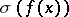 
have the same degree and if 
is irreducible over the field of fractions of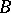 
, then there is no factorization 
where 
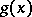and 
and 
are not constant. For example, a polynomial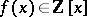 
with leading coefficient 1 is prime in 
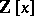(hence irreducible in 
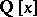) if for some prime 
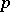the polynomial 
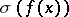obtained from 
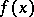by reducing the coefficients modulo 
is irreducible.
References
| [1] | B.L. van der Waerden, "Algebra" , 1–2 , Springer (1967–1971) (Translated from German) |
| [2] | S. Lang, "Algebra" , Addison-Wesley (1974) |
| [3] | O. Zariski, P. Samuel, "Commutative algebra" , 1–2 , Springer (1975) |
Comments
A factorial ring is also known as a unique factorization domain (UFD).
Irreducible polynomial. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Irreducible_polynomial&oldid=31615