Convex hull
of a set 
The minimal convex set containing  ; it is the intersection of all convex sets containing
; it is the intersection of all convex sets containing 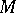 . The convex hull of a set
. The convex hull of a set  is denoted by
is denoted by 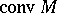 . In the Euclidean space
. In the Euclidean space 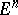 the convex hull is the set of possible locations of the centre of gravity of a mass which can be distributed in
the convex hull is the set of possible locations of the centre of gravity of a mass which can be distributed in  in different manners. Each point of the convex hull is the centre of gravity of a mass concentrated at not more than
in different manners. Each point of the convex hull is the centre of gravity of a mass concentrated at not more than 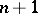 points (Carathéodory's theorem).
points (Carathéodory's theorem).
The closure of the convex hull is called the closed convex hull. It is the intersection of all closed half-spaces containing 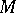 or is identical with
or is identical with 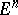 . The part of the boundary of the convex hull not adjacent to
. The part of the boundary of the convex hull not adjacent to 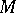 has the local structure of a developable hypersurface. In
has the local structure of a developable hypersurface. In  the convex hull of a bounded closed set
the convex hull of a bounded closed set 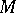 is the convex hull of the extreme points of
is the convex hull of the extreme points of 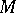 (an extreme point of
(an extreme point of  is a point of this set which is not an interior point of any segment belonging to
is a point of this set which is not an interior point of any segment belonging to 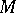 ).
).
In addition to Euclidean spaces, convex hulls are usually considered in locally convex linear topological spaces 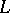 . In
. In 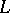 , the convex hull of a compact set
, the convex hull of a compact set  is the closed convex hull of its extreme points (the Krein–Mil'man theorem).
is the closed convex hull of its extreme points (the Krein–Mil'man theorem).
References
| [1] | R.E. Edwards, "Functional analysis: theory and applications" , Holt, Rinehart & Winston (1965) |
| [2] | R.R. Phelps, "Lectures on Choquet's theorem" , v. Nostrand (1966) |
Convex hull. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Convex_hull&oldid=31501