Bessel functions

Cylinder functions of the first kind. A Bessel function of order can be defined as the series

(*)

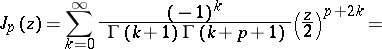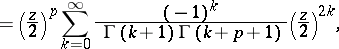which converges throughout the plane. A Bessel function of order is the solution of the corresponding Bessel equation. If the argument and the order are real numbers, the Bessel function is real, and its graph has the form of a damped vibration (Fig.); if the order is even, the Bessel function is even, if odd, it is odd.

Figure: b015840a

Graphs of the functions and .

The behaviour of a Bessel function in a neighbourhood of zero is given by the first term of the series (*); for large , the asymptotic representation

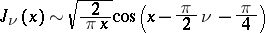holds. The zeros of a Bessel function (i.e. the roots of the equation ) are simple, and the zeros of are situated between the zeros of . Bessel functions of "half-integral" order are expressible by trigonometric functions; in particular

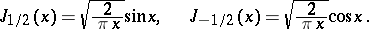The Bessel functions 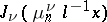(where are the positive zeros of , ) form an orthogonal system with weight in the interval . Under certain conditions the following expansion is valid:

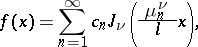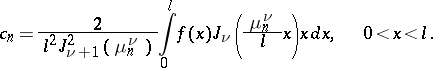In an infinite interval this expansion is replaced by the Fourier–Bessel integral

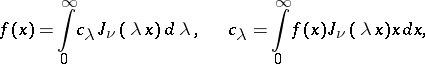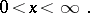The following formulas play an important role in the theory of Bessel functions and their applications:

1) the integral representation

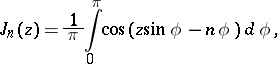2) the generating function

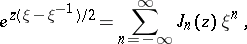3) the addition theorem for Bessel functions of order zero

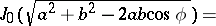4) the recurrence formulas

For references, see Cylinder functions.