Uniform convergence
of a sequence of functions (mappings)
A property of a sequence  , where
, where 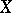 is an arbitrary set,
is an arbitrary set, 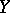 is a metric space,
is a metric space, 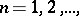 converging to a function (mapping)
converging to a function (mapping) 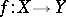 , requiring that for every
, requiring that for every 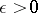 there is a number
there is a number 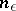 (independent of
(independent of  ) such that for all
) such that for all 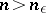 and all
and all 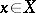 the inequality
the inequality
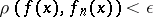 |
holds. This is equivalent to
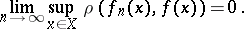 |
In order that a sequence 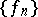 converges uniformly on a set
converges uniformly on a set 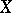 to a function
to a function 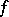 it is necessary and sufficient that there is a sequence of numbers
it is necessary and sufficient that there is a sequence of numbers 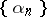 such that
such that 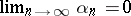 , as well as a number
, as well as a number 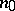 such that for
such that for  and all
and all 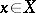 the inequality
the inequality
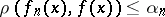 |
holds.
Example. The sequence 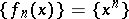 ,
, 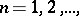 converges uniformly on any interval
converges uniformly on any interval 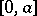 ,
, 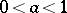 , but does not converge uniformly on
, but does not converge uniformly on  .
.
A necessary and sufficient condition for uniform convergence that does not use the limit function is given by the Cauchy criterion for uniform convergence.
Properties of uniformly-convergent sequences.
1) If 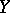 is a normed linear space and two sequences of mappings
is a normed linear space and two sequences of mappings 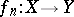 and
and 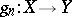 converge uniformly on
converge uniformly on 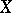 , then for any
, then for any 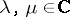 the sequence
the sequence 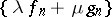 also converges uniformly on
also converges uniformly on 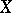 .
.
2) If 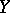 is a linear normed ring, if the sequence
is a linear normed ring, if the sequence 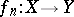 ,
,  is uniformly convergent on
is uniformly convergent on 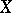 and if
and if 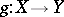 is a bounded mapping, then the sequence
is a bounded mapping, then the sequence  also converges uniformly on
also converges uniformly on 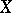 .
.
3) If 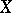 is a topological space,
is a topological space, 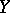 is a metric space and if a sequence of mappings
is a metric space and if a sequence of mappings  , continuous at
, continuous at 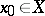 , converges uniformly on
, converges uniformly on 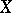 to
to 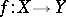 , then
, then 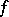 is also continuous at
is also continuous at 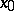 , that is,
, that is,
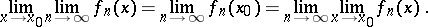 |
The condition of uniform convergence of the sequence  on
on 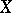 is essential in this result, in the sense that there are sequences of numerical functions, continuous on an interval, that converge at all points to a function that is not continuous on the interval in question. An example is
is essential in this result, in the sense that there are sequences of numerical functions, continuous on an interval, that converge at all points to a function that is not continuous on the interval in question. An example is 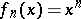 ,
, 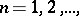 on
on 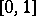 . Uniform convergence of a sequence of continuous functions is not a necessary condition for continuity of the limit function. However, if
. Uniform convergence of a sequence of continuous functions is not a necessary condition for continuity of the limit function. However, if  is a compact set,
is a compact set,  is the set of real numbers
is the set of real numbers 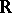 and if all functions in a sequence of continuous functions
and if all functions in a sequence of continuous functions 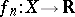 simultaneously increase or decrease at all points
simultaneously increase or decrease at all points 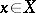 and the sequence has a finite limit:
and the sequence has a finite limit:
 |
then in order that 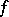 be continuous on
be continuous on 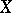 it is necessary and sufficient that
it is necessary and sufficient that  converges uniformly on that set. Necessary, and simultaneously sufficient, conditions for the continuity of the limit of a sequence of continuous functions in general are given in terms of quasi-uniform convergence of the sequence.
converges uniformly on that set. Necessary, and simultaneously sufficient, conditions for the continuity of the limit of a sequence of continuous functions in general are given in terms of quasi-uniform convergence of the sequence.
4) If a sequence of Riemann- (Lebesgue-) integrable functions 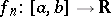 ,
, 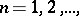 converges uniformly on
converges uniformly on 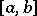 to a function
to a function 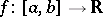 , then this function is also Riemann- (respectively, Lebesgue-) integrable, for any
, then this function is also Riemann- (respectively, Lebesgue-) integrable, for any 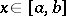 one has
one has
 | (*) |
and the convergence of the sequence 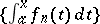 to
to 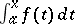 is uniform on
is uniform on 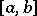 . Formula (*) has been generalized to the case of a Stieltjes integral. If, however, a sequence of integrable functions
. Formula (*) has been generalized to the case of a Stieltjes integral. If, however, a sequence of integrable functions 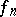 ,
,  on
on 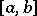 only converges at each point of the interval to an integrable function
only converges at each point of the interval to an integrable function 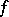 , then (*) need not hold.
, then (*) need not hold.
5) If a sequence of continuously differentiable functions 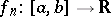 ,
, 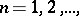 on
on 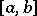 converges at some point
converges at some point 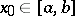 and if the sequence of derivatives
and if the sequence of derivatives 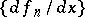 converges uniformly on
converges uniformly on 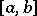 , then the sequence
, then the sequence 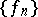 also converges uniformly on
also converges uniformly on 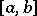 , its limit is a continuously differentiable function on the interval and
, its limit is a continuously differentiable function on the interval and
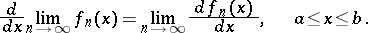 |
Let 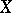 be a set and
be a set and 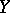 a metric space. A family of functions (mappings)
a metric space. A family of functions (mappings) 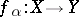 ,
, 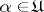 , with
, with  a topological space, is said to be uniformly convergent as
a topological space, is said to be uniformly convergent as 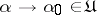 to the function (mapping)
to the function (mapping) 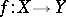 if for every
if for every 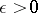 there is a neighbourhood
there is a neighbourhood  of
of 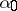 such that for all
such that for all  and
and  the inequality
the inequality
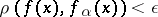 |
holds.
For uniformly-convergent families of functions there are properties similar to the above-mentioned properties of uniformly-convergent sequences of functions.
The concept of uniform convergence of mappings can be generalized to the case when 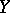 is a uniform space, in particular, when
is a uniform space, in particular, when  is a topological group.
is a topological group.
References
| [1] | P.S. Aleksandrov, "Einführung in die Mengenlehre und die allgemeine Topologie" , Deutsch. Verlag Wissenschaft. (1984) (Translated from Russian) |
| [2] | A.N. Kolmogorov, S.V. Fomin, "Elements of the theory of functions and functional analysis" , 1–2 , Graylock (1957–1961) (Translated from Russian) |
| [3] | J.L. Kelley, "General topology" , Springer (1975) |
Comments
The theorem that a monotone sequence of continuous functions converges uniformly to its pointwise limit if this limit is continuous, is known as Dini's theorem.
References
| [a1] | R. Engelking, "General topology" , Heldermann (1989) |
| [a2] | W. Rudin, "Principles of mathematical analysis" , McGraw-Hill (1976) pp. 75–78 |
Uniform convergence. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Uniform_convergence&oldid=30905