Subgroup series

A finite chain of subgroups of a group 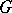contained in each other:

(*)

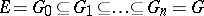or

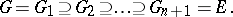One also considers infinite chains of imbedded subgroups (increasing and decreasing), which may be indexed by a sequence of numbers or even by elements of an ordered set. They are often called subgroup systems (cf. Subgroup system).

An important part in group theory is played by subnormal, normal and central series. A subgroup series (*) is called subnormal if each group in the chain is a normal subgroup of the subsequent term. If also each subgroup 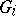, , 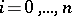is normal in 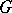, the series (*) is called a normal series in 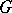. There is also a different terminology, in which the name normal series is given to what is here called subnormal, while for the second concept defined here one uses the term "invariant subgroup seriesinvariant series" . The quotient groups 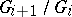are called factors, while the number is the length of the subnormal series. A normal series (*) is called central if all its factors are central, i.e. 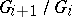lies in the centre of the group for all 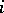, or, which is equivalent, the commutator of and 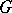lies in 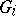for all 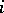. If is the centre of the group 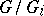(respectively, if the commutator of 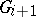and 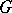coincides with 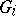) for all 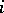, then the series (*) is called the upper central series (respectively, the lower central series) of 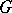. Let a subnormal (respectively, normal or central) series be given in a group together with a certain subgroup , and let 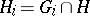, 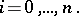Then the chain

is a subnormal (respectively, normal or central) series in , while the factors of this series are isomorphic to subgroups of the corresponding factors in the series (*). If 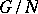is a quotient group of 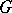, the chain

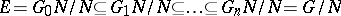is a subnormal (respectively, normal or central) series in 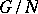, and the factors in this series are homomorphic images of the corresponding factors in the series (*).

Two subnormal (in particular, normal) series in a group are said to be isomorphic if they have the same length and if there is a bijection between their factors such that corresponding factors are isomorphic. If every subgroup of one of the series coincides with one of the subgroups of the other, the second series is called a refinement of the first. A normal series which cannot be refined is called a principal series (or chief series); while a subnormal series which cannot be refined is called a composition series. The factors in these series are called chief and composition factors, respectively. Any two subnormal (respectively, normal or central) series of a group have isomorphic subnormal (respectively, normal or central) refinements. In particular, any two chief (composition) series are isomorphic (see Jordan–Hölder theorem).

References

[1]	M.I. Kargapolov, J.I. [Yu.I. Merzlyakov] Merzljakov, "Fundamentals of the theory of groups" , Springer (1979) (Translated from Russian)
[2]	S.N. Chernikov, "Groups with given properties of subgroup systems" , Moscow (1980) (In Russian)

References

[a1]	M. Suzuki, "Group theory" , 1 , Springer (1986)
[a2]	D.J.S. Robinson, "A course in the theory of groups" , Springer (1980)