Figure
A subset  of a homogeneous space
of a homogeneous space 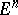 with fundamental group
with fundamental group  that can be included in a system
that can be included in a system 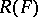 of subsets of this space isomorphic to some space of a geometric object
of subsets of this space isomorphic to some space of a geometric object 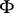 (see Geometric objects, theory of).
(see Geometric objects, theory of). 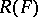 is called the figure space of
is called the figure space of 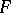 . The components of
. The components of 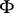 are called the coordinates of the associated figure
are called the coordinates of the associated figure 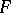 . To each figure
. To each figure 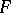 in
in  corresponds a class
corresponds a class 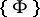 of similar geometric objects. The rank, genre, characteristic, and type of a geometric object
of similar geometric objects. The rank, genre, characteristic, and type of a geometric object 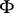 in
in 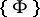 are called the rank, genre, characteristic, and type of the figure
are called the rank, genre, characteristic, and type of the figure 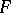 (the so-called arithmetic invariants of the figure, cf. [2]). For example, a circle in three-dimensional Euclidean space is a figure of rank 6, genre 1, characteristic 1, and type 1; a point in three-dimensional projective space is a figure of rank 3, genre 0, characteristic 2, and type 1. The completely-integrable system of Pfaffian equations defining the geometric object
(the so-called arithmetic invariants of the figure, cf. [2]). For example, a circle in three-dimensional Euclidean space is a figure of rank 6, genre 1, characteristic 1, and type 1; a point in three-dimensional projective space is a figure of rank 3, genre 0, characteristic 2, and type 1. The completely-integrable system of Pfaffian equations defining the geometric object 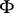 is called the stationarity system of equations of
is called the stationarity system of equations of 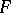 .
.
Let 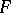 and
and 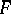 be two figures in
be two figures in 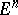 . If there is a mapping of
. If there is a mapping of 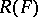 onto
onto 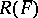 under which every geometric object corresponding to
under which every geometric object corresponding to 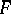 is covered by every geometric object corresponding to
is covered by every geometric object corresponding to 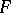 , then one says that
, then one says that 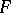 covers or induces
covers or induces 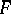 (
(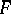 is said to be covered or induced by
is said to be covered or induced by 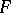 ). A figure
). A figure 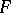 of rank
of rank 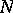 is called simple if it does not cover any other figure of lower rank.
is called simple if it does not cover any other figure of lower rank. 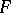 is called an inducing figure of index
is called an inducing figure of index 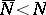 if there is a figure of rank
if there is a figure of rank 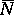 that is covered by
that is covered by 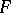 , while the rank
, while the rank 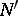 of any other figure
of any other figure 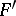 covered by
covered by 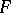 does not exceed
does not exceed 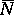 . For example, a point, a
. For example, a point, a 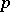 -dimensional plane and a hyperquadric in an
-dimensional plane and a hyperquadric in an  -dimensional projective space are simple figures, and a hyperquadric in an
-dimensional projective space are simple figures, and a hyperquadric in an  -dimensional affine space and a
-dimensional affine space and a  -dimensional
-dimensional 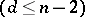 quadric in an
quadric in an  -dimensional projective space are inducing figures of indices
-dimensional projective space are inducing figures of indices  and
and 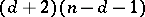 , respectively.
, respectively.
An ordered set of two figures, 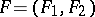 , is called a figure pair. The incidence coefficient of a figure pair is the number
, is called a figure pair. The incidence coefficient of a figure pair is the number 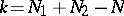 , where
, where 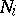 (
(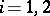 ) is the rank of
) is the rank of 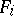 , and
, and 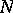 is the rank of the system of forms
is the rank of the system of forms 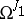 ,
, 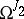 ,
, 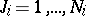 , that are the left-hand sides of the stationarity equations of
, that are the left-hand sides of the stationarity equations of 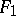 and
and 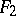 . If
. If  , then the pair
, then the pair 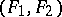 is called non-incident.
is called non-incident.
References
| [1] | G.F. Laptev, "Differential geometry of imbedded manifolds. Group-theoretical method of differential-geometric investigation" Trudy Moskov. Mat. Obshch. , 2 (1953) pp. 275–383 (In Russian) |
| [2] | V.S. Malakhovskii, "Differential geometry of manifolds of figures and of figure pairs" Trudy Geom. Sem. Inst. Nauchn. Inform. Akad. Nauk SSSR , 2 (1969) pp. 179–206 (In Russian) |
| [3] | V.S. Malakhovskii, "Differential geometry of lines and surfaces" J. Soviet Math. , 2 (1974) pp. 304–330 Itogi Nauk. i Tekhn. Algebra. Topol. Geom. , 10 (1972) pp. 113–158 |
Figure. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Figure&oldid=30777