Bilinear form

on a product of modules

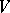A bilinear mapping , where is a left unitary -module, 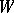is a right unitary 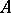-module, and 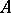is a ring with a unit element, which is also regarded as an -bimodule. If 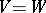, one says that is a bilinear form on the module , and also that 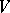has a metric structure given by 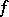. Definitions involving bilinear mappings make sense also for bilinear forms. Thus, one speaks of the matrix of a bilinear form with respect to chosen bases in 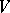and 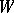, of the orthogonality of elements and submodules with respect to bilinear forms, of orthogonal direct sums, of non-degeneracy, etc. For instance, if 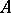is a field and 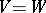is a finite-dimensional vector space over 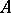with basis , then for the vectors

and

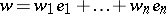the value of the form will be

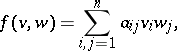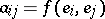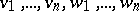where . The polynomial in the variables is sometimes identified with 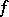and is called a bilinear form on 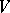. If the ring 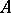is commutative, a bilinear form is a special case of a sesquilinear form (with the identity automorphism).

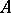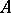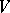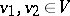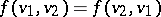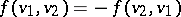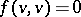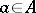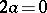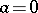Let be a commutative ring. A bilinear form on an -module is said to be symmetric (or anti-symmetric or skew-symmetric) if for all one has (or ), and is said to be alternating if . An alternating bilinear form is anti-symmetric; the converse is true only if for any it follows from that . If 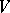has a finite basis, symmetric (or anti-symmetric or alternating) forms on and only such forms have a symmetric (anti-symmetric, alternating) matrix in this basis. The orthogonality relation with respect to a symmetric or anti-symmetric form on is symmetric.

A bilinear form 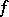on 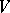is said to be isometric with a bilinear form 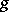on 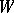if there exists an isomorphism of 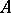-modules 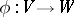such that

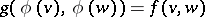for all 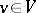. This isomorphism is called an isometry of the form and, if 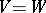and 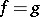, a metric automorphism of the module 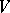(or an automorphism of the form 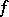). The metric automorphisms of a module form a group (the group of automorphisms of the form 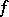); examples of such groups are the orthogonal group or the symplectic group.

Let 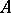be a skew-field and let 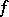be a bilinear form on 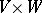; let the spaces 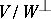and 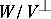be finite-dimensional over 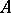; one then has

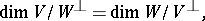and this number is called the rank of . If 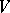is finite-dimensional and is non-degenerate, then

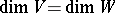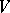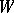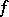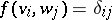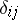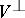and for each basis in there exists a basis in which is dual with respect to ; it is defined by the condition , where are the Kronecker symbols. If, in addition, , then the submodules and 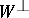are said to be the right and the left kernel of 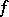, respectively; for symmetric and anti-symmetric forms the right and left kernels are identical and are simply referred to as the kernel.

Let 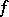be a symmetric or an anti-symmetric bilinear form on 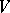. An element 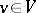for which 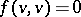is said to be an isotropic element; a submodule is said to be isotropic if , and totally isotropic if 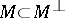. Totally isotropic submodules play an important role in the study of the structure of bilinear forms (cf. Witt decomposition; Witt theorem; Witt ring). See also Quadratic form for the structure of bilinear forms.

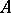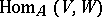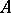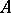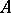Let be commutative, let be the -module of all -linear mappings from into , and let be the -module of all bilinear forms on 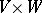. For every bilinear form 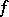on 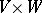and for each 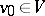, the formula

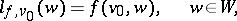defines an 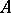-linear form on 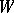. Correspondingly, for 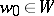the formula

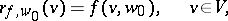defines an -linear form on 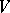. The mapping 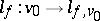is an element of

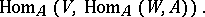The mapping 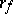in

is defined in a similar way. The mappings 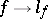and define isomorphisms between the 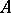-modules

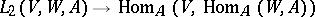and

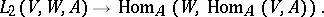A bilinear form is said to be left-non-singular (respectively, right-non-singular) if 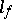(respectively, 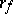) is an isomorphism; if 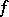is both left- and right-non-singular, it is said to be non-singular; otherwise it is said to be singular. A non-degenerate bilinear form may be singular. For free modules 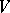and 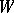of the same finite dimension a bilinear form 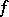on 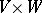is non-singular if and only if the determinant of the matrix of 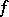with respect to any bases in 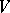and is an invertible element of the ring . The following isomorphisms

and

given by a non-singular bilinear form 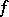, are defined by the formulas

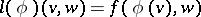and

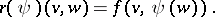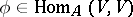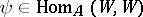The endomorphisms and are said to be conjugate with respect to the form 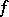if .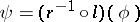

References

[1]	N. Bourbaki, "Elements of mathematics. Algebra: Algebraic structures. Linear algebra" , 1 , Addison-Wesley (1974) pp. Chapt.1;2 (Translated from French)
[2]	S. Lang, "Algebra" , Addison-Wesley (1974)
[3]	E. Artin, "Geometric algebra" , Interscience (1957)