Sesquilinear form

A function in two variables on a module (for example, on a vector space) which is linear in one variable and semi-linear in the other. More precisely, a sesquilinear form on a unitary module over an associative-commutative ring with an identity, equipped with an automorphism , is a mapping , , linear in for fixed , and semi-linear in for fixed (see Semi-linear mapping). Analogously one defines a sesquilinear mapping 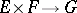, whe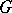re , , are -modules. In the case when 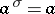(), one obtains the notion of a bilinear form (or a bilinear mapping). Another important example of a sesquilinear form is obtained when 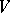is a vector space over the field and . Special cases of sesquilinear forms are Hermitian forms (cf. Hermitian form) (and also skew-Hermitian forms).

Sesquilinear forms can also be considered on modules over a non-commutative ring 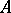; in this case it is assumed that 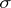is an anti-automorphism, that is,

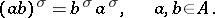For sesquilinear forms it is possible to introduce many notions of the theory of bilinear forms, for example the notions of an orthogonal submodule, a left and a right kernel, a non-degenerate form, the matrix of the form in a given basis, the rank of the form, and conjugate homomorphisms.

References

[1]	N. Bourbaki, "Algèbre" , Eléments de mathématiques , 2 , Hermann (1942–1959)
[2]	S. Lang, "Algebra" , Addison-Wesley (1984)

Comments

Let 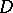be a division ring with centre and a right vector space over 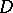. Let be an anti-automorphism of , i.e. is an automorphism of the underlying additive group of and . A sesquilinear form relative to on is a bi-additive mapping

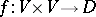such that

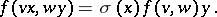Unless , the anti-automorphism is obviously uniquely determined by .

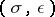Let . A -Hermitian form is a sesquilinear form on such that moreover

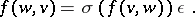One must then have and for all . The concepts of a Hermitian, anti-Hermitian, symmetric, anti-symmetric, or bilinear form (or matrix) for complex vector spaces (with complex conjugation) arise as the special cases of a 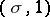-Hermitian form, a -Hermitian form, an -Hermitian form, and an Hermitian form.

Given a subspace , 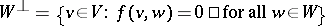. A subspace 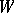is totally isotropic if . The Witt index of a sesquilinear form is the dimension of a maximal totally-isotropic subspace.

References

[a1]	J. Tits, "Buildings and BN-pairs of spherical type" , Springer (1974) pp. Chapt. 8
[a2]	J.A. Dieudonné, "La géométrie des groups classiques" , Springer (1963)