Semi-definite form

A quadratic form over an ordered field which represents either only non-negative field elements or only non-positive field elements. In the former case the form is said to be a non-negative definite ( for all ), in the latter case — a non-positive definite quadratic form (). Most frequently one considers semi-definite forms over the field of real numbers. For the field a similar definition yields the concept of (non-negative and non-positive) semi-definite Hermitian quadratic forms (see Hermitian form).

If is a symmetric bilinear (cf. Bilinear form) or Hermitian form such that is a semi-definite form, then is sometimes also called a (non-negative or non-positive) semi-definite form. If is a quadratic or Hermitian semi-definite form in a vector space 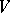, then 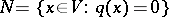is a subspace, identical with the kernel of 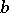, and the given form naturally induces a positive-definite or negative-definite form on .

Comments

Instead of "non-negative definite" one also says positive semi-definite, and instead of "non-positive definite" also negative semi-definite.