Additive arithmetic function

An arithmetic function of one argument that satisfies the following conditions for two relatively prime integers

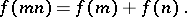An additive arithmetic function is said to be strongly additive if 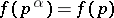for all prime numbers 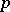and all positive integers 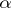. An additive arithmetic function is said to be completely additive if the condition 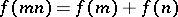is satisfied for relatively non-prime integers 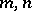as well; in such a case .

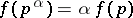Examples. The function 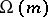, which is the number of all prime divisors of the number (multiple divisors are counted according to their multiplicity), is an additive arithmetic function; the function , which is the number of different prime divisors of the number 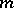, is strongly additive; and the function 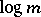is completely additive.

Comments

An arithmetic function is also called a number-theoretic function.