Absolutely integrable function

A function such that its absolute value is integrable. If a function is Riemann-integrable on the segment $[a,b]$, $a<b$, its absolute value is also Riemann-integrable on this interval, and

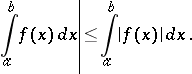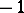A similar assertion is also valid for a function of variables which is Riemann-integrable on a cube-filled domain in the -dimensional Euclidean space. The converse theorem for Riemann-integrable functions is not true: The function which is equal to 1 for rational values of and is equal to for irrational values of is not Riemann-integrable, but its absolute value is integrable. The situation is different for Lebesgue-integrable functions: A Lebesgue-measurable function 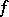is Lebesgue-integrable (Lebesgue-summable) on a measurable set 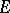in the -dimensional space if and only if its absolute value is Lebesgue-integrable on this set. The following inequality is valid:

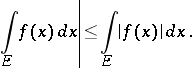In the case of improper one-dimensional Riemann or Lebesgue integrals on a half-open interval , 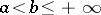(provided that the function 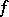is, respectively, Riemann-integrable or Lebesgue-integrable on any segment 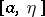, 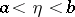), the existence of the improper integral of the absolute value of the function,

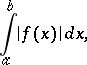implies the existence of the integral

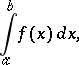but the converse statement is not true (cf. Absolutely convergent improper integral). In this connection it should be noted that, if the improper integral

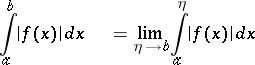exists, then 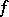is Lebesgue-integrable on , and its improper integral is equal to the Lebesgue integral.

In the case of functions of several (more than one) variables, improper integrals are usually so defined that the existence of the improper integral of the absolute value of a function is equivalent to the existence of the improper integral of the function itself.

Let the values of a function 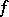be in some Banach space with norm 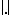. The function is then called absolutely integrable on a measurable set 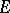if the integral

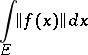exists; also, if 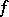is integrable on 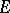, the relationship

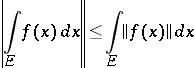is true.

References

[1]	V.A. Il'in, E.G. Poznyak, "Fundamentals of mathematical analysis" , 1–2 , MIR (1982) (Translated from Russian)
[2]	L.D. Kudryavtsev, "Mathematical analysis" , 1 , Moscow (1973) (In Russian) MR1617334 MR1070567 MR1070566 MR1070565 MR0866891 MR0767983 MR0767982 MR0628614 MR0619214 Zbl 1080.00002 Zbl 1080.00001 Zbl 1060.26002 Zbl 0869.00003 Zbl 0696.26002 Zbl 0703.26001 Zbl 0609.00001 Zbl 0632.26001 Zbl 0485.26002 Zbl 0485.26001
[3]	S.M. Nikol'skii, "A course of mathematical analysis" , 1 , MIR (1977) (Translated from Russian) Zbl 0397.00003 Zbl 0384.00004
[4]	L. Schwartz, "Cours d'analyse" , 1 , Hermann (1967) MR0756815 MR0756814

References

[a1]	H.L. Royden, "Real analysis" , Macmillan (1968) MR1013117 MR1532990 MR0151555 Zbl 1191.26002 Zbl 0704.26006 Zbl 0197.03501 Zbl 0121.05501
[a2]	A.C. Zaanen, "Integration" , North-Holland (1967) MR0222234 Zbl 0175.05002
[a3]	W. Rudin, "Principles of mathematical analysis" , McGraw-Hill (1953) MR0055409 Zbl 0052.05301
[a4]	W. Rudin, "Real and complex analysis" , McGraw-Hill (1966) pp. 98 MR0210528 Zbl 0142.01701
[a5]	A.E. Taylor, "General theory of functions and integration" , Blaisdell (1965) MR0178100 Zbl 0135.11301
[a6]	C.D. Aliprantz, O. Burleinshaw, "Principles of real analysis" , North-Holland (1981)