Young criterion
From Encyclopedia of Mathematics
One of the sufficiency criteria for the convergence of a Fourier series at a point. Let a function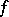 
have period 
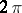, be Lebesgue integrable over the interval 
and, on putting 
, let it satisfy at the point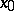 
the conditions: 1) 
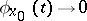as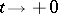 
; 2) the function 
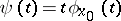is of finite variation 
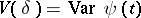on the interval 
, 
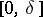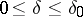, where 
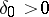is some fixed number; and 3) 
as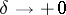 
. Then the Fourier series of 
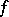at 
converges to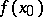 
(cf. [2]). Young's criterion is stronger than the Jordan criterion. It was established by W.H. Young [1].
References
| [1] | W.H. Young, "On the convergence of the derived series of Fourier series" Proc. London Math. Soc. , 17 (1916) pp. 195–236 |
| [2] | N.K. [N.K. Bari] Bary, "A treatise on trigonometric series" , Pergamon (1964) (Translated from Russian) |
Comments
References
| [a1] | A. Zygmund, "Trigonometric series" , 2 , Cambridge Univ. Press (1988) |
How to Cite This Entry:
Young criterion. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Young_criterion&oldid=28452
Young criterion. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Young_criterion&oldid=28452
This article was adapted from an original article by B.I. Golubov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article