Favard measure

of order of a subset in a Euclidean space of dimension

A generalization of the Hausdorff measure; it was introduced by J. Favard [1]. The precise definition is: The group of motions of induces on the collection of its -dimensional affine subspaces a left-invariant Haar measure that is unique up to normalization, and the set induces a function the value of which at an affine subspace is the number of points in the intersection 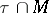. The Favard measure of 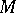is the value of at 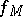, if the normalizing constant is chosen so that 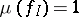for the 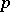-dimensional unit cube .

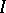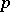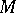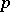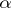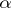The Favard measure of order of a set does not exceed its Hausdorff -measure and, in the case when , it is the same as if and only if 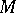splits into a countable number of parts one of which has Hausdorff 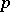-measure zero and each of the others can be situated on a smooth 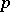-dimensional manifold.

References

[1]	J. Favard, "Une définition de la longueur et de l'aire" C.R. Acad. Sci. Paris , 194 (1932) pp. 344–346
[2]	H. Federer, "Geometric measure theory" , Springer (1969)