Elliptic partial differential equation
at a given point 
A partial differential equation of order  ,
,
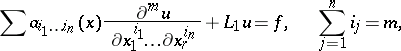 |
such that  is a differential operator of order less than
is a differential operator of order less than  , whose characteristic equation at
, whose characteristic equation at  ,
,
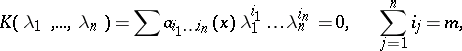 |
has no real roots except  .
.
For second-order equations the characteristic form is quadratic,
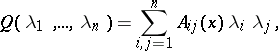 |
and can be brought to the form
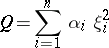 |
by a non-singular affine transformation of the variables 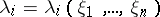 ,
,  .
.
When all  or all
or all 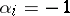 , the equation is said to be of elliptic type.
, the equation is said to be of elliptic type.
A partial differential equation is said to be of elliptic type in its domain of definition if it is elliptic at every point of this domain.
An elliptic partial differential is called uniformly elliptic if there are positive numbers  and
and  such that
such that
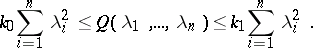 |
For references see Differential equation, partial.
Comments
References
| [a1] | L.V. Hörmander, "The analysis of linear partial differential operators" , 1 , Springer (1983) |
Elliptic partial differential equation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Elliptic_partial_differential_equation&oldid=28185