Diffusion equation

A second-order partial differential equation describing the process of diffusion, i.e. the process of equalization of the concentration in a medium with an initially non-homogeneous distribution of some substance. The diffusion equation has the form

(1)

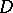where is the porosity coefficient, is the diffusion coefficient and 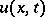is the concentration of the substance at a point of the medium at the moment of time 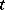. The diffusion equation is derived by making up the balance of the substance using Nerst's diffusion law. It is assumed in so doing that sources of the substance and diffusion into an external medium are absent in the domain under consideration. Such a diffusion equation is said to be homogeneous. If the domain under consideration contains sources of the substance with a volume distribution density 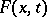, the diffusion process is said to be inhomogeneous with right-hand side 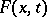. If the substance falls apart or multiplies at a rate proportional to the initial concentration, a term should be inserted in the right-hand side of the diffusion equation.

The diffusion equation is of parabolic type. In order to find a unique solution, initial and boundary conditions are imposed. The initial condition for a diffusion equation is to specify the concentration 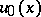of the substance at the initial moment of time:

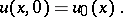(2)

If the substance then fills the entire space, one obtains the Cauchy problem (1), (2). If, on the other hand, the diffusion substance occupies a volume 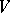bounded by the side surface 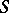, as well as the initial condition (2), a boundary condition is imposed on 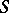. The three fundamental linear boundary conditions for a diffusion equation are listed below.

1) The concentration of the substance 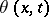is specified on 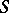; then

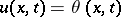is a boundary condition of the first kind.

2) The density 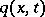of the flow of the substance entering 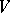through 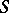is given; then

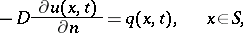where is the interior normal to the surface 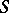, is a boundary condition of the second kind (if is impermeable, ).

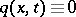3) If 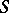is semi-permeable, and if the diffusion taking place into the external medium with a given concentration through 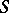is linear, then

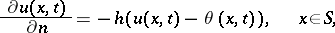is a boundary condition of the third kind.

Other boundary conditions, including non-linear ones, may also be imposed on 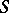, as well as conditions involving derivatives of a higher order than those which appear in the diffusion equation. Since a diffusion equation is a special case of a differential equation describing physical equalization processes, it is analogous to the thermal-conductance equation, the Navier–Stokes equations for the laminar flow of an incompressible liquid, the equation of pure electric conductance, etc.

References

[1]	A.N. [A.N. Tikhonov] Tichonoff, A.A. Samarskii, "Differentialgleichungen der mathematischen Physik" , Deutsch. Verlag Wissenschaft. (1959) (Translated from Russian)

Comments

For the role of diffusion equations in the theory of stochastic processes see Diffusion process.

Another notation for the diffusion equation is

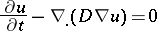(where, of course, 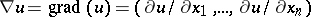and 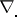is another notation for ).

The non-linear equation (in one space variable)

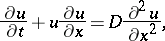where 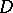is a constant, is referred to as the one-dimensional non-linear diffusion equation or the Burgers equation. The monograph [a3] is devoted to this equation.

References

[a1]	J. Crank, "The mathematics of diffusion" , Clarendon Press (1956)
[a2]	H.S. Carslaw, J.C. Jaeger, "Conduction of heat in solids" , Clarendon Press (1959)
[a3]	J.M. Burgers, "The nonlinear diffusion equation" , Reidel (1974)