Jacobian

Jacobi determinant

A determinant of a matrix of special form whose entries are first-order partial derivatives of functions. Let 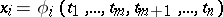, , 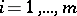be given functions having first-order partial derivatives with respect to the variables 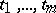. The Jacobian of these functions is the determinant

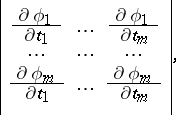which for brevity is denoted by the symbol

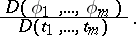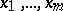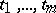The modulus of a Jacobian characterizes the dilatation (contraction) of the volume element in the transition from the variables to . The name is given after C.G.J. Jacobi, who first studied its properties and applications.

References

[1]	V.A. Il'in, E.G. Poznyak, "Fundamentals of mathematical analysis" , 1–2 , MIR (1982) (Translated from Russian)
[2]	L.D. Kudryavtsev, "Mathematical analysis" , 1–2 , Moscow (1973) (In Russian)
[3]	S.M. Nikol'skii, "A course of mathematical analysis" , 2 , MIR (1977) (Translated from Russian)

Comments

The above Jacobian is also denoted as

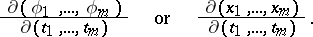The entry at place 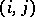(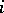-th row, 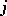-th column) is . The matrix with these entries is also called a Jacobian matrix (not to be confused with a Jacobi matrix). The Jacobian plays a role in the statement of the inverse function theorem and in change-of-variable formulas for integrals and differential forms.

References

[a1]	M. Spivak, "Calculus on manifolds" , Benjamin/Cummings (1965)
[a2]	W. Rudin, "Principles of mathematical analysis" , McGraw-Hill (1953)