Borel function

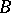-function

A function for which all subsets of the type 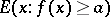in its domain of definition are Borel sets (cf. Borel set). Such functions are also known as Borel-measurable functions, or 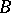-measurable functions. The operations of addition, multiplication and limit transition — as in the general case of measurable functions — do not take one outside the class of Borel functions, but, unlike in the general case, the superposition of two Borel functions does also not lead outside the class of Borel functions. Moreover, [1], if is a measurable function on an arbitrary space and if 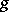is a Borel function on the space of real numbers, then the function is measurable on . All Borel functions are Lebesgue-measurable (cf. Measurable function). The converse proposition is not true. However, for any Lebesgue-measurable function 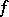there exists a Borel function 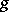such that 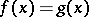almost-everywhere [1]. Borel functions are sometimes called Baire functions, since the set of all Borel functions is identical with the set of functions belonging to the Baire classes (Lebesgue's theorem, [2]). Borel functions can be classified by the order of the Borel sets 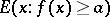; the classes thus obtained are identical with the Baire classes.

The concept of a Borel function has been generalized to include functions with values in an arbitrary metric space [3]. One then also speaks of 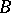-measurable mappings. Borel functions have found use not only in set theory and function theory but also in probability theory [1], [4].

References

[1]	P.R. Halmos, "Measure theory" , v. Nostrand (1950)
[2]	F. Hausdorff, "Grundzüge der Mengenlehre" , Leipzig (1914) (Reprinted (incomplete) English translation: Set theory, Chelsea (1978))
[3]	K. Kuratowski, "Topology" , 1–2 , Acad. Press (1966–1968) (Translated from French)
[4]	A.N. Kolmogorov, "Foundations of the theory of probability" , Chelsea, reprint (1950) (Translated from German)