Absolute continuity
Absolute continuity of an integral as a property of the (Lebesgue) integral. Let a function 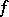 be
be 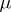 -integrable on a set
-integrable on a set 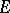 . The integral of
. The integral of 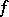 over
over 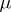 -measurable subsets
-measurable subsets  is an absolutely continuous set function (see Subsection 3 below) with respect to the measure
is an absolutely continuous set function (see Subsection 3 below) with respect to the measure  if for any
if for any  there exists a
there exists a 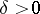 such that the integral
such that the integral 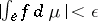 for any set
for any set  with
with 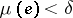 . In the general case the integral with respect to a finitely-additive set function with scalar or vectorial
. In the general case the integral with respect to a finitely-additive set function with scalar or vectorial 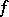 or
or 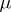 is an absolutely continuous function.
is an absolutely continuous function.
A.P. TerekhinV.F. Emel'yanov
Absolute continuity of a measure as a concept in the theory of measures. A measure  is absolutely continuous with respect to a measure
is absolutely continuous with respect to a measure 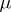 if
if  is an absolutely continuous set function with respect to
is an absolutely continuous set function with respect to 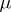 . Thus, let
. Thus, let  be a finite measure, given together with
be a finite measure, given together with 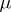 on some fixed
on some fixed 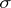 -algebra
-algebra 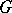 ;
;  will then be absolutely continuous with respect to
will then be absolutely continuous with respect to 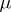 if it follows from
if it follows from 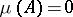 ,
, 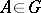 , that
, that 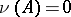 . A generalized finite measure (cf. Charge)
. A generalized finite measure (cf. Charge)  is absolutely continuous with respect to a generalized measure
is absolutely continuous with respect to a generalized measure 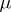 if
if 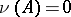 , provided that
, provided that 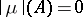 , where
, where  is the total variation of
is the total variation of 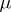 .
.
A.P. Terekhin
Absolute continuity of a function is a stronger notion than continuity. A function  defined on a segment
defined on a segment 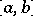 is said to be absolutely continuous if for any
is said to be absolutely continuous if for any 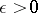 there exists a
there exists a 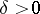 such that for any finite system of pairwise non-intersecting intervals
such that for any finite system of pairwise non-intersecting intervals 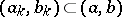 ,
, 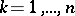 , for which
, for which
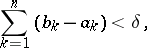 |
the inequality
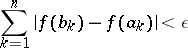 |
holds. Any absolutely continuous function on a segment is continuous on this segment. The opposite implication is not true: e.g. the function 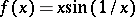 if
if 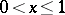 and
and  is continuous on the segment
is continuous on the segment 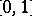 , but is not absolutely continuous on it. If, in the definition of an absolutely continuous function, the requirement that the pairwise intersections of intervals
, but is not absolutely continuous on it. If, in the definition of an absolutely continuous function, the requirement that the pairwise intersections of intervals 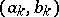 are empty be discarded, then the function will satisfy an even stronger condition: A Lipschitz condition with some constant.
are empty be discarded, then the function will satisfy an even stronger condition: A Lipschitz condition with some constant.
If two functions 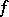 and
and 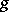 are absolutely continuous, then their sum, difference and product are also absolutely continuous and, if
are absolutely continuous, then their sum, difference and product are also absolutely continuous and, if  does not vanish, so is their quotient
does not vanish, so is their quotient 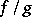 . The superposition of two absolutely continuous functions need not be absolutely continuous. However, if the function
. The superposition of two absolutely continuous functions need not be absolutely continuous. However, if the function 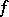 is absolutely continuous on a segment
is absolutely continuous on a segment 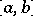 and if
and if 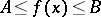 ,
, 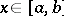 , while the function
, while the function 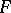 satisfies a Lipschitz condition on the segment
satisfies a Lipschitz condition on the segment 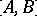 , then the composite function
, then the composite function 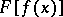 is absolutely continuous on
is absolutely continuous on  . If a function
. If a function 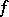 , which is absolutely continuous on
, which is absolutely continuous on  , is monotone increasing, while
, is monotone increasing, while 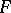 is absolutely continuous on
is absolutely continuous on  , then the function
, then the function 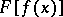 is also absolutely continuous on
is also absolutely continuous on 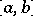 .
.
An absolutely continuous function maps a set of measure zero into a set of measure zero, and a measurable set into a measurable set. Any continuous function of finite variation which maps each set of measure zero into a set of measure zero is absolutely continuous. Any absolutely continuous function can be represented as the difference of two absolutely continuous non-decreasing functions.
A function 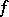 that is absolutely continuous on the segment
that is absolutely continuous on the segment 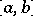 has a finite variation on this segment and has a finite derivative
has a finite variation on this segment and has a finite derivative 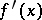 at almost every point. The derivative
at almost every point. The derivative 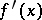 is summable over this segment, and
is summable over this segment, and
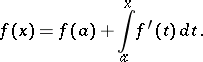 |
If the derivative of an absolutely continuous function is almost everywhere equal to zero, then the function itself is constant. On the other hand, for any function  that is summable on
that is summable on 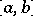 the function
the function 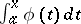 is absolutely continuous on this segment. Accordingly, the class of functions that are absolutely continuous on a given segment coincides with the class of functions that can be represented as an indefinite Lebesgue integral, i.e. as a Lebesgue integral with a variable upper limit of a certain summable function plus a constant.
is absolutely continuous on this segment. Accordingly, the class of functions that are absolutely continuous on a given segment coincides with the class of functions that can be represented as an indefinite Lebesgue integral, i.e. as a Lebesgue integral with a variable upper limit of a certain summable function plus a constant.
If 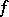 is absolutely continuous on
is absolutely continuous on 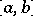 , then its total variation is
, then its total variation is
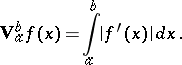 |
The concept of absolute continuity can be generalized to include both functions of several variables and set functions (see Subsection 4 below).
References
| [1] | A.N. Kolmogorov, S.V. Fomin, "Elements of the theory of functions and functional analysis" , 1–2 , Graylock (1957–1961) (Translated from Russian) |
| [2] | V.I. Smirnov, "A course of higher mathematics" , 5 , Addison-Wesley (1964) (Translated from Russian) |
L.D. Kudryavtsev
Absolute continuity of a set function is a concept usually applied to countably-additive functions defined on a 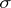 -ring
-ring 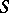 of subsets of a set
of subsets of a set 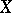 . Thus, if
. Thus, if 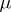 and
and  are two countably-additive functions defined on
are two countably-additive functions defined on 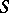 having values in the extended real number line
having values in the extended real number line  , then
, then  is absolutely continuous with respect to
is absolutely continuous with respect to 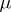 (in symbols this is written as
(in symbols this is written as 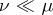 ) if
) if 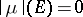 entails
entails  . Here
. Here 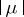 is the total variation of
is the total variation of 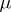 :
:
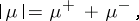 |
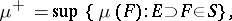 |
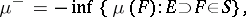 |
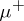 and
and 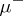 are measures, known as the positive and negative variations of
are measures, known as the positive and negative variations of  ; according to the Jordan–Hahn theorem,
; according to the Jordan–Hahn theorem, 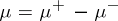 . It turns out that the relations 1)
. It turns out that the relations 1) 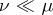 ; 2)
; 2) 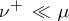 ,
, 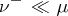 ; 3)
; 3) 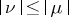 are equivalent. If the measure
are equivalent. If the measure  is finite,
is finite, 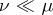 if and only if for any
if and only if for any 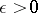 there exists a
there exists a  such that
such that  entails
entails 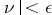 . According to the Radon–Nikodým theorem, if
. According to the Radon–Nikodým theorem, if 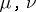 are (completely)
are (completely) 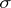 -finite, (i.e.
-finite, (i.e.  and there exists a sequence
and there exists a sequence 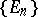 ,
, 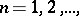 such that
such that
 |
and if 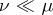 , then there exists on
, then there exists on 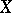 a finite measurable function
a finite measurable function 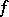 such that
such that
 |
Conversely, if 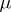 is (completely)
is (completely) 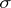 -finite and the integral
-finite and the integral 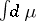 makes sense, then
makes sense, then 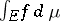 as a function of the set
as a function of the set  is absolutely continuous with respect to
is absolutely continuous with respect to  . If
. If 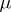 and
and  are (completely)
are (completely)  -finite measures on
-finite measures on 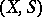 , there exist uniquely defined (completely)
, there exist uniquely defined (completely) 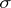 -finite measures
-finite measures 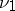 and
and 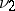 such that
such that 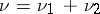 ,
, 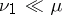 and
and  is singular with respect to
is singular with respect to 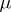 (i.e. there exists a set
(i.e. there exists a set 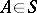 such that
such that 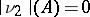 ,
, 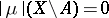 ) (Lebesgue's theorem). A measure, defined on the Borel sets of a finite-dimensional Euclidean space (or, more generally, of a locally compact group), is called absolutely continuous if it is absolutely continuous with respect to the Lebesgue (Haar) measure. A non-negative measure
) (Lebesgue's theorem). A measure, defined on the Borel sets of a finite-dimensional Euclidean space (or, more generally, of a locally compact group), is called absolutely continuous if it is absolutely continuous with respect to the Lebesgue (Haar) measure. A non-negative measure 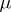 on the Borel sets of the real line is absolutely continuous if and only if the corresponding distribution function
on the Borel sets of the real line is absolutely continuous if and only if the corresponding distribution function 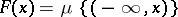 is absolutely continuous (as a function of a real variable). The concept of absolute continuity of a set function can also be defined for finitely-additive functions and for functions with vector values.
is absolutely continuous (as a function of a real variable). The concept of absolute continuity of a set function can also be defined for finitely-additive functions and for functions with vector values.
References
| [1] | P.R. Halmos, "Measure theory" , v. Nostrand (1950) |
| [2] | J. Neveu, "Bases mathématiques du calcul des probabilités" , Masson (1970) |
V.V. Sazonov
Comments
References
| [a1] | H.L. Royden, "Real analysis" , Macmillan (1968) |
| [a2] | A.C. Zaanen, "Integration" , North-Holland (1967) |
| [a3] | W. Rudin, "Principles of mathematical analysis" , McGraw-Hill (1953) |
| [a4] | W. Rudin, "Real and complex analysis" , McGraw-Hill (1966) pp. 98 |
| [a5] | A.E. Taylor, "General theory of functions and integration" , Blaisdell (1965) |
| [a6] | C.D. Aliprantz, O. Burleinshaw, "Principles of real analysis" , North-Holland (1981) |
Absolute continuity. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Absolute_continuity&oldid=27231