Topological ring

A ring 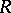that is a topological space, such that the mappings

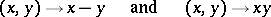are continuous. A topological ring 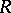is called separated if it is separated as a topological space (cf. Separation axiom). In this case 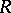is a Hausdorff space. Any subring of a topological ring , and also the quotient ring 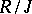by an ideal 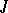, is a topological ring. If 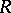is separated and the ideal 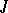is closed, then is a separated topological ring. The closure 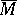of a subring 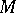in is also a topological ring. A direct product of topological rings is a topological ring in a natural way.

A homomorphism of topological rings is a ring homomorphism which is also a continuous mapping. If 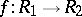is such a homomorphism, where 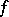is moreover an epimorphism and an open mapping, then 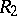is isomorphic as a topological ring to 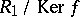. Banach algebras are an example of topological rings. An important type of topological ring is defined by the property that it has a fundamental system of neighbourhoods of zero consisting of some set of ideals. For example, to any ideal 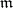in a commutative ring one can associate the 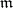-adic topology, in which the sets 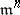for all natural numbers form a fundamental system of neighbourhoods of zero. This topology is separated if the condition

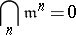is satisfied.

For a topological ring 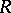one can define its completion , which is a complete topological ring, and a separated topological ring 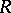can be imbedded as an everywhere-dense subset in 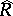, which is also separated in this case. The additive group of the ring 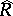coincides with the completion of the additive group of 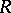, as an Abelian topological group.

References

[1]	N. Bourbaki, "Elements of mathematics. General topology" , Addison-Wesley (1966) (Translated from French)
[2]	N. Bourbaki, "Elements of mathematics. Commutative algebra" , Addison-Wesley (1972) (Translated from French)
[3]	L.S. Pontryagin, "Topological groups" , Princeton Univ. Press (1958) (Translated from Russian)
[4]	B.L. van der Waerden, "Algebra" , 1–2 , Springer (1967–1971) (Translated from German)