Lacunary sequence
From Encyclopedia of Mathematics
A sequence of numbers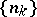 
such that 
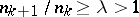; this class of sequences is denoted by 
and is used, in particular, in the theory of lacunary series and in the theory of lacunary trigonometric series. There are generalizations of the class 
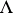. For example, the class 
: 
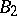if there is an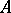 
such that the number of solutions of the equations 
(
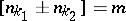and 
is the integer part of the number 
) does not exceed 
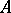for any integer 
; the class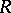 
: 
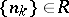if there is an 
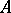such that the number of solutions of the equations 
(
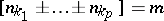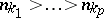) does not exceed 
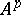for any 
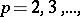and any integer 
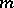; and the classes 
, 
, 
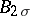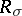, consisting of sequences that split into finitely-many sequences of the classes 
, 
, 
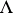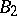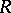, respectively.
References
| [1] | N.K. [N.K. Bari] Bary, "A treatise on trigonometric series" , Pergamon (1964) (Translated from Russian) |
How to Cite This Entry:
Lacunary sequence. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lacunary_sequence&oldid=25904
Lacunary sequence. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lacunary_sequence&oldid=25904
This article was adapted from an original article by V.F. Emel'yanov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article