Spectral density, estimator of the
A function of the observed values  of a discrete-time stationary stochastic process, used as an estimator of the spectral density
of a discrete-time stationary stochastic process, used as an estimator of the spectral density  . As an estimator of the spectral density one often uses quadratic forms
. As an estimator of the spectral density one often uses quadratic forms
 |
where the  are complex coefficients (depending on
are complex coefficients (depending on  ). It can be shown that the asymptotic behaviour as
). It can be shown that the asymptotic behaviour as  of the first two moments of an estimator of the spectral density is satisfactory, in general, if one considers only the subclass of quadratic forms such that
of the first two moments of an estimator of the spectral density is satisfactory, in general, if one considers only the subclass of quadratic forms such that  when
when  . This enables one to restrict attention to estimators of the spectral density of the form
. This enables one to restrict attention to estimators of the spectral density of the form
 |
where
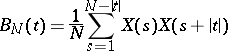 |
is a sample estimator of the covariance function of the stationary process  and the
and the  are suitably chosen weights. The estimator
are suitably chosen weights. The estimator  can be written as
can be written as
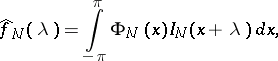 |
where  is the periodogram and
is the periodogram and 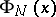 is some continuous even function with
is some continuous even function with 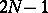 of its Fourier coefficients specified:
of its Fourier coefficients specified:
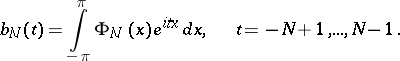 |
The function 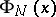 is called a spectral window; one usually considers spectral windows of the form
is called a spectral window; one usually considers spectral windows of the form
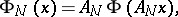 |
where 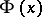 is some continuous function on
is some continuous function on  such that
such that
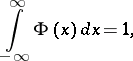 |
and 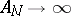 as
as 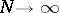 , but
, but  . Similarly, one considers coefficients
. Similarly, one considers coefficients  of the form
of the form
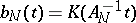 |
and a function  , called a lag window or covariance window. Under weak smoothness restrictions on the spectral density
, called a lag window or covariance window. Under weak smoothness restrictions on the spectral density  , or assuming that
, or assuming that  is mixing, it is possible to prove that for a wide class of spectral or covariance windows the estimator
is mixing, it is possible to prove that for a wide class of spectral or covariance windows the estimator 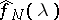 is asymptotically unbiased and consistent.
is asymptotically unbiased and consistent.
In the case of a multi-dimensional stochastic process, estimation of the elements of the matrix of spectral densities  proceeds in a similar way using the corresponding periodogram
proceeds in a similar way using the corresponding periodogram 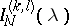 . Instead of an estimator of the spectral density in the form of a quadratic form in the observations, one often assumes that the spectral density depends in a particular way on a finite number of parameters, and then one seeks estimators based on the observations of the parameters involved in this expression for the spectral density (see Maximum-entropy spectral estimator; Spectral estimator, parametric).
. Instead of an estimator of the spectral density in the form of a quadratic form in the observations, one often assumes that the spectral density depends in a particular way on a finite number of parameters, and then one seeks estimators based on the observations of the parameters involved in this expression for the spectral density (see Maximum-entropy spectral estimator; Spectral estimator, parametric).
References
| [1] | D.R. Brillinger, "Time series. Data analysis and theory" , Holt, Rinehart & Winston (1975) |
| [2] | E.J. Hannan, "Multiple time series" , Wiley (1972) |
| [3] | T.M. Anderson, "Statistical analysis of time series" , Wiley (1971) |
Comments
References
| [a1] | G.E.P. Box, G.M. Jenkins, "Time series analysis. Forecasting and control" , Holden-Day (1960) |
| [a2] | P.E. Caines, "Linear stochastic systems" , Wiley (1988) |
| [a3] | K.O. Dzhaparidze, "Parameter estimation and hypothesis testing in spectral analysis of stationary time series" , Springer (1986) |
| [a4] | L. Ljung, "System identification theory for the user" , Prentice-Hall (1987) |
Spectral density, estimator of the. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Spectral_density,_estimator_of_the&oldid=24129