Brauer group

of a field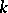

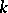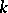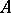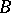The group of classes of finite-dimensional central simple algebras (cf. Central simple algebra) over with respect to the equivalence defined as follows. Two central simple -algebras and of finite rank are equivalent if there exist positive integers 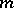and such that the tensor products 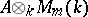and 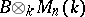are isomorphic 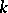-algebras (here is the algebra of square matrices of order over 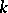). The tensor multiplication of algebras induces an Abelian group structure on the set of equivalence classes of finite-dimensional central simple algebras. This group is also known as the Brauer group of and is denoted by . 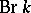The zero element of this group is the class of full matrix algebras, while the element inverse to the class of an algebra is the class of its opposite algebra. Each non-zero class contains, up to isomorphism, exactly one division algebra over 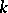(i.e. a skew-field over ).

Brauer groups were defined and studied in several publications by R. Brauer, E. Noether, A. Albert, H. Hasse and others, starting in the 1920s (see, for example, [6]). The most complete results, including the computation of the Brauer group, were obtained for number fields in connection with the development of class field theory. The general form of the reciprocity law is formulated in terms of Brauer groups.

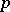The Brauer group is zero for any separably-closed field and any finite field. For the field of real numbers the Brauer group is a cyclic group of order two and its non-zero element is the class of the quaternion algebra. If is the field of -adic numbers or any locally compact field that is complete with respect to a discrete valuation, then its Brauer group is isomorphic to 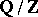, where 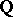is the additive group of rational numbers and 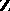is the additive group of integers. This fact is of importance in local class-field theory.

Let 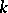be an algebraic number field of finite degree or a field of algebraic functions in one variable with a finite field of constants. Then there exists an exact sequence of groups

where runs through all possible norms of the field 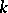, 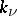are the respective completions of 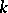, and the homomorphism inv is induced by the natural imbeddings . The image of an element from 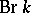in 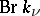is called a local invariant, the homomorphism 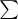is the summation of local invariants. This fact is established in global class-field theory.

If 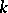is a field of algebraic functions in one variable over an algebraically closed field of constants, then its Brauer group is zero (Tsen's theorem). The case of an arbitrary field of constants is treated in [4] and in [7].

The Brauer group depends functorially on 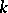, i.e. if 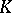is an extension of the field 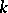, a homomorphism 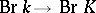is defined. Its kernel, denoted by 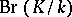, consists of classes of algebras splitting over .

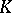The construction of cross products with the aid of factor systems [5] results in a cohomological interpretation of Brauer groups. For any normal extension 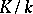there exists an isomorphism

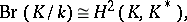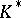where is the second Galois cohomology group with coefficients in the multiplicative group of 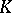. Moreover, the group 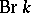is isomorphic to 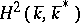, where is the separable closure of 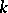. A central simple algebra is assigned its class in the Brauer group by the coboundary operator

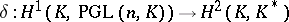in the cohomology sequence corresponding to the exact group sequence

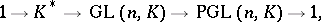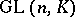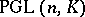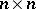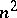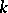where and are the linear and the projective matrix groups of order . Here the set is interpreted as the set of -isomorphism classes of central simple algebras of rank over the field which split over , or as the set of classes of 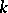-isomorphic Brauer–Severi varieties of dimension , possessing a point that is rational over 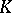(cf. Brauer–Severi variety).

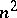All Brauer groups are periodic groups. The order of any of its elements is a divisor of the number , where is the rank of the skew-field representing this element.

The cohomological interpretation of the Brauer group makes it possible to consider it as the group of classes of extensions of the Galois group of the separable closure 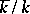by the group .

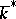A generalization of the concept of a Brauer group is the Brauer–Grothendieck group, whose definition is analogous to that of the Brauer group, except that the central simple algebras are replaced by Azumaya algebras [7].

References

[1]	J.W.S. Cassels (ed.) A. Fröhlich (ed.) , Algebraic number theory , Acad. Press (1967)
[2]	N. Bourbaki, "Elements of mathematics. Algebra: Algebraic structures. Linear algebra" , 1 , Addison-Wesley (1974) pp. Chapt.1;2 (Translated from French)
[3]	J.-P. Serre, "Cohomologie Galoisienne" , Springer (1964)
[4]	D.K. Faddeev, "On the theory of algebras over fields of algebraic functions in one variable" Vestnik Leningrad. Univ. : 7 (1957) pp. 45–51 (In Russian) (English summary)
[5]	N.G. Chebotarev, "Introduction to the theory of algebra..." , Moscow-Leningrad (1949) (In Russian)
[6]	M. Deuring, "Algebren" , Springer (1935)
[7]	A. Grothendieck, "Le groupe de Brauer I, II, III" A. Grothendieck (ed.) J. Giraud (ed.) et al. (ed.) , Dix exposés sur la cohomologie des schémas , North-Holland & Masson (1968) pp. 46–188
[8]	J.S. Milne, "Etale cohomology" , Princeton Univ. Press (1980)

Comments

For the construction of cross products with the aid of factor systems see also Cross product; Extension of a group. The latter article contains the notion of a system of factors.

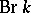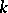A recent result in the theory of Brauer groups is a theorem of Merkuryev and Suslin [a1], which in its simplest form asserts that is generated by the classes of algebras that split over a cyclic extension of , provided that is a field of characteristic zero containing all roots of unity. The proof is based on the close relationship between the theory of Brauer groups and algebraic 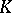-theory. An algebra 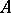over a commutative ring is an Azumaya algebra if it is finitely generated and central over and separable.

References

[a1]	A. Suslin, "Plenary adress" A.M. Gleason (ed.) , Proc. Internat. Congress Mathematicians (Berkeley, 1986) , Amer. Math. Soc. (1987) pp. 1195–1209