Normal analytic space

An analytic space the local rings of all points of which are normal, that is, are integrally-closed integral domains. A point of an analytic space is said to be normal (one also says that is normal at ) if the local ring is normal. In a neighbourhood of such a point the space has a reduced and irreducible model. Every simple (non-singular) point is normal. The simplest example of a normal analytic space is an analytic manifold.

In what follows the (complete non-discretely normed) ground field is assumed to be algebraically closed. In this case the most complete results on normal analytic spaces have been obtained (see [1]) and a normalization theory has been constructed [2] that gives a natural link between arbitrary reduced analytic spaces and normal analytic spaces. Let be the set of points of an analytic space that are not normal and let 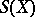be the set of singular points of 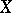(cf. Singular point). Then:

1) 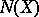and 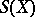are closed analytic subspaces of 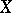, and ;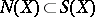

2) for ,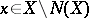

(that is, a normal analytic space is smooth in codimension 1);

3) if 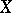is a complete intersection at and if the above inequality holds, then 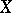is normal at that point.

A normalization of a reduced analytic space 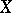is a pair 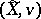, where 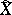is a normal analytic space and 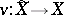is a finite surjective analytic mapping inducing an isomorphism of the open sets

The normalization is uniquely determined up to an isomorphism, that is, if 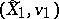and 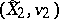are two normalizations,

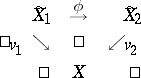then there exists a unique analytic isomorphism such that the diagram commutes. The normalization exists and has the following properties. For every point the set of irreducible components of 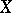at is in one-to-one correspondence with 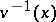. The fibre at 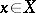of the direct image of the structure sheaf is naturally isomorphic to the integral closure of the ring 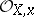in its complete ring of fractions.

The concept of a normal analytic space over 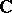can be introduced in terms of analytic continuation of holomorphic functions [3]. Namely, a reduced complex space is normal if and only if Riemann's first theorem on the removal of singularities holds for it: If 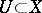is an open subset and 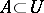is a closed analytic subset not containing irreducible components of 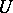, then any function that is holomorphic on and locally bounded on 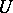has a unique analytic continuation to a holomorphic function on 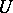. For normal complex spaces Riemann's second theorem on the removal of singularities also holds: If 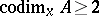at every point 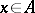, then the analytic continuation in question is possible without the requirement that the function is bounded. A reduced complex space 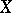is normal if and only if for every open set 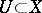the restriction mapping of holomorphic functions

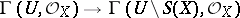is bijective. The property of being normal can also be phrased in the language of local cohomology — it is equivalent to 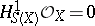(see [5]). For any reduced complex space 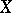one can define the sheaf of rings of germs of weakly holomorphic functions, that is, functions satisfying the conditions of Riemann's first theorem. It turns out that the ring 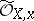is finite as an 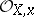-module and equal to the integral closure of 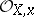in its complete ring of fractions. In other words, 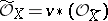, where 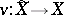is the normalization mapping.

A normal complex space can also be characterized in the following manner: A complex space is normal if and only if every point of it has a neighbourhood that admits an analytic covering onto a domain of 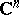(see [3], [8]).

A reduced complex space 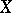is a Stein space if and only if its normalization has this property (see [4]). To normal complex spaces one can extend the concept of a Hodge metric (see Kähler metric). Kodaira's projective imbedding theorem [6] carries over to compact normal spaces with such a metric.

In algebraic geometry one examines analogues of normal analytic spaces: normal algebraic varieties (see Normal scheme). For algebraic varieties over a complete non-discretely normed field the two concepts are the same (see [7], [1]).

References

[1]	S.S. Abhyankar, "Local analytic geometry" , Acad. Press (1964)
[2]	C. Houzel, "Géometrie analytique locale I" , Sem. H. Cartan Ann. 13 1960/61 , 2 (1963) pp. Exp. 18–21
[3]	H. Grauert, R. Remmert, "Komplexe Räume" Math. Ann. , 136 (1958) pp. 245–318
[4]	R. Narasimhan, "A note on Stein spaces and their normalisations" Ann. Scuola Norm. Sup. Pisa , 16 (1962) pp. 327–333
[5]	Y.T. Siu, G. Trautmann, "Gap sheaves and extensions of coherent analytic subsheaves" , Springer (1971)
[6]	H. Grauert, "Ueber Modifikationen und exzeptionelle analytische Mengen" Math. Ann. , 146 (1962) pp. 331–368
[7]	O. Zariski, P. Samuel, "Commutative algebra" , 2 , Springer (1960)
[8]	B.A. Fuks, "Theory of analytic functions of several complex variables" , 1 , Amer. Math. Soc. (1963) (Translated from Russian)

References

[a1]	H. Whitney, "Complex analytic varieties" , Addison-Wesley (1972) pp. Chapt. 8