Gel'fand representation

A mapping establishing a correspondence between an element of a commutative Banach algebra and a function on the space of maximal ideals of . There exists a one-to-one correspondence between the points of and the homomorphisms of into the field of complex numbers. If the corresponding identification is made, the Gel'fand representation is realized by the formula . In the special case of the group algebra of a locally compact Abelian group (with convolution taken as multiplication in the algebra, cf. also Group algebra of a locally compact group) the Gel'fand representation coincides with the Fourier transform (for more details see Banach algebra). The Gel'fand transform was introduced by I.M. Gel'fand [1].

References

[1]	I.M. Gel'fand, "Normierte Ringe" Mat. Sb. , 9 (51) : 1 (1941) pp. 3–24

Comments

The Gel'fand representation is also called the Gel'fand transform, cf. [a2] and Commutative Banach algebra.

Using the Gel'fand representations of specially chosen algebras one can prove various approximation theorems (cf., e.g., [a2], Sect. 11.13). A well-known such theorem is Wiener's theorem (cf. also [a1], Chapt. XI, Sect. 2): If is a non-vanishing absolutely-convergent (Fourier) series on the interval , then 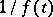can be represented as an absolutely-convergent Fourier series on this interval.

In algebraic geometry a very similar representation/transform is used. Let 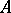be a commutative ring with unity. To an element 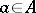one associates the morphism of affine schemes 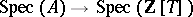(function) given by the ring homomorphism 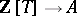, 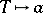(cf. Affine scheme). In the case of affine varieties over an algebraically closed field 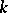, the function 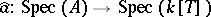, where now 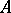is a -algebra, takes the value 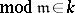at the closed point of 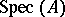represented by the maximal ideal , showing the relationship of this construction with the Gel'fand transform.

References

[a1]	K. Yosida, "Functional analysis" , Springer (1980) pp. Chapt. 8, Sect. 4; 5
[a2]	W. Rudin, "Functional analysis" , McGraw-Hill (1979)